Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

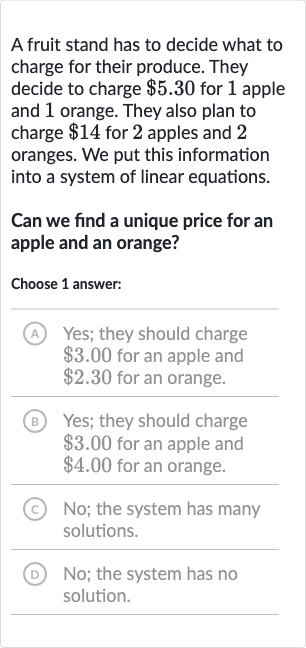
A fruit stand has to decide what to charge for their produce. They decide to charge $5.30 for 1 apple and 1 orange. They also plan to charge $14 for 2 apples and 2 oranges. We put this information into a system of linear equations. Can we find a unique price for an apple and an orange? Choose 1 answer: (A) Yes; they should charge $3.00 for an apple and $2.30 for an orange. (B) Yes; they should charge $3.00 for an apple and $4.00 for an orange. (c) No; the system has many solutions. (D) No; the system has no solution.

A fruit stand has to decide what to charge for their produce. They decide to charge $5.30 \$ 5.30 for 11 apple and 11 orange. They also plan to charge $14 \$ 14 for 22 apples and 22 oranges. We put this information into a system of linear equations.\newlineCan we find a unique price for an apple and an orange?\newlineChoose 11 answer:\newline(A) Yes; they should charge $3.00 \$ 3.00 for an apple and $2.30 \$ 2.30 for an orange.\newlineB Yes; they should charge $3.00 \$ 3.00 for an apple and $4.00 \$ 4.00 for an orange.\newline(C) No; the system has many solutions.\newline(D) No \mathrm{No} ; the system has no solution.

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Solve a system of equations using any method: word problemsright chevron icon

Full solution

Q. A fruit stand has to decide what to charge for their produce. They decide to charge $5.30 \$ 5.30 for 11 apple and 11 orange. They also plan to charge $14 \$ 14 for 22 apples and 22 oranges. We put this information into a system of linear equations.\newlineCan we find a unique price for an apple and an orange?\newlineChoose 11 answer:\newline(A) Yes; they should charge $3.00 \$ 3.00 for an apple and $2.30 \$ 2.30 for an orange.\newlineB Yes; they should charge $3.00 \$ 3.00 for an apple and $4.00 \$ 4.00 for an orange.\newline(C) No; the system has many solutions.\newline(D) No \mathrm{No} ; the system has no solution.
  1. Equations Setup: Let's denote the price of an apple as AA and the price of an orange as OO. We can then write the given information as a system of linear equations:\newline11. For 11 apple and 11 orange, the cost is $5.30\$5.30:\newlineA+O=5.30A + O = 5.30\newline22. For 22 apples and 22 oranges, the cost is $14.00\$14.00:\newline2A+2O=14.002A + 2O = 14.00
  2. Equations Simplification: We can simplify the second equation by dividing every term by 22 to make it easier to compare with the first equation:\newline2A+2O=14.002A + 2O = 14.00\newlineA+O=7.00A + O = 7.00\newlineNow we have two equations:\newline11. A+O=5.30A + O = 5.30\newline22. A+O=7.00A + O = 7.00
  3. Inconsistency Identified: We can see that the two equations are inconsistent with each other because they both describe a relationship between AA and OO, but they give different sums for the same pair of variables. This means that there is no unique solution to this system of equations.
  4. Final Answer: Since the system of equations does not have a unique solution, the correct answer is:\newline(C) No; the system has many solutions.

More problems from Solve a system of equations using any method: word problems

Question
Solve using substitution. 5x−2y=−7 5x - 2y = -7 x=−5 x = -5 (_,_)
Get tutor helpright-arrow

Posted 5 months ago

Question
Is (1,1)(1,1) a solution to this system of equations? \newline4x+10y=144x + 10y = 14\newlinex+6y=7x + 6y = 7\newlineChoices:\newline(A) yes \newline(B) no
Get tutor helpright-arrow

Posted 5 months ago

Question
Which describes the system of equations below?\newliney=–3x+9y = –3x + 9 \newliney=–3x+9y = –3x + 9 \newlineChoices:\newline(A) consistent and independent\text{(A) consistent and independent} \newline(B) consistent and dependent\text{(B) consistent and dependent} \newline(C) inconsistent\text{(C) inconsistent}
Get tutor helpright-arrow

Posted 5 months ago

Question
Solve using elimination. \newline7x−8y=−17 7x - 8y = -17 \newline −7x+3y=2 -7x + 3y = 2 \newline (____,____)(\_\_\_\_, \_\_\_\_)
Get tutor helpright-arrow

Posted 5 months ago

Question
Solve. \newlinex=−2 x = -2 \newline−2x+2y=−8 -2x + 2y = -8 \newline(____,____)(\_\_\_\_, \_\_\_\_)
Get tutor helpright-arrow

Posted 9 months ago

Question
Write a system of equations to describe the situation below, solve using any method, and fill in the blanks.\newlineAt a community barbecue, Mrs. Wilkerson and Mr. Hogan are buying dinner for their families. Mrs. Wilkerson purchases 33 hot dog meals and 33 hamburger meals, paying a total of $36\$36. Mr. Hogan buys 11 hot dog meal and 33 hamburger meals, spending $26\$26 in all. How much do the meals cost?\newlineHot dog meals cost $\$_______ each, and hamburger meals cost $\$________ each.
Get tutor helpright-arrow

Posted 9 months ago

Question
Solve the system of equations by substitution.\newline−3x−y−3z=−11-3x - y - 3z = -11\newlinez=5z = 5\newlinex−y+3z=19x - y + 3z = 19\newline(____.____,____)
Get tutor helpright-arrow

Posted 5 months ago

Question
Solve the system of equations by elimination.\newlinex−3y−2z=10 x - 3y - 2z = 10 \newline3x+2y+2z=14 3x + 2y + 2z = 14 \newline2x−3y−2z=16 2x - 3y - 2z = 16 \newline(_,_,_)(\_,\_,\_)
Get tutor helpright-arrow

Posted 5 months ago

Question
Solve the system of equations.\newliney=x2+36x+3 y = x^2 + 36x + 3 \newliney=22x−37 y = 22x - 37 \newlineWrite the coordinates in exact form. Simplify all fractions and radicals.\newline(_,_)(\_,\_)\newline(_,_)(\_,\_)
Get tutor helpright-arrow

Posted 5 months ago

Question
Solve the system of equations.\newliney=−x−24 y = -x - 24 \newlinex2+y2=488 x^2 + y^2 = 488 \newlineWrite the coordinates in exact form. Simplify all fractions and radicals.\newline(_,_) (\_,\_) \newline(_,_) (\_,\_)
Get tutor helpright-arrow

Posted 5 months ago

View all (92)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant