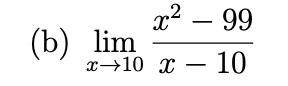AI tutor
Full solution
Q. Evaluate
- Identify Problem Type: Identify the type of problem.We are asked to find the limit of a rational function as approaches a specific value. This is a limit problem in calculus.
- Substitute Value: Substitute the value of into the function to see if the function is defined at that point.We encounter a division by zero, which means the function is not defined at , and we have an indeterminate form of type .
- Factor Numerator: Factor the numerator to simplify the expression.The numerator can be factored as because it is a difference of squares.
- Cancel Common Factors: Cancel out the common factors in the numerator and the denominator.The term in the numerator cancels with the term in the denominator, simplifying the expression to:
- Substitute Simplified Function: Substitute the value of into the simplified function.Now that the indeterminate form has been resolved, we can substitute directly into the simplified function: