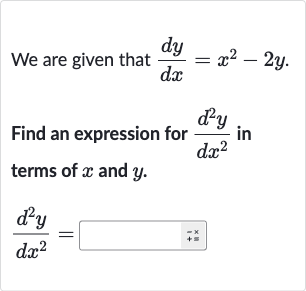
We are given that (dy)/(dx)=x^(2)-2y. Find an expression for (d^(2)y)/(dx^(2)) in terms of x and y. (d^(2)y)/(dx^(2))=

Question

We are given that  (dy)/(dx)=x^(2)-2y. Find an expression for  (d^(2)y)/(dx^(2)) in terms of  x and  y.  (d^(2)y)/(dx^(2))=

Bytelearn · Accepted Answer

Given First Derivative: We are given the first derivative (dy)/(dx) = x^2 - 2y. To find the second derivative (d^(2)y)/(dx^(2)), we need to differentiate (dy)/(dx) with respect to x.Apply Chain Rule: Using the chain rule, we differentiate (dy)/(dx) with respect to x. The chain rule states that the derivative of y with respect to x is the derivative of y with respect to an intermediate variable (like u) times the derivative of that intermediate variable with respect to x. (d^(2)y)/(dx^(2)) = d/dx(x^2) - d/dx(2y)Differentiate Terms: Differentiate x^2 with respect to x to get 2x. (d^(2)y)/(dx^(2)) = 2x - d/dx(2y)Product Rule Application: To differentiate 2y with respect to x, we use the fact that (dy)/(dx) = x^2 - 2y. We apply the product rule to the constant 2 and the function y. (d^(2)y)/(dx^(2)) = 2x - 2*(dy)/(dx)Substitute Expression: Substitute the expression for (dy)/(dx) into the equation. (d^(2)y)/(dx^(2)) = 2x - 2*(x^2 - 2y)Simplify Expression: Simplify the expression by distributing the -2 and combining like terms. (d^(2)y)/(dx^(2)) = 2x - 2x^2 + 4yFinal Second Derivative: The final expression for the second derivative in terms of x and y is: (d^(2)y)/(dx^(2)) = -2x^2 + 2x + 4y

We are given that $\frac{d y}{d x}=x^{2}-2 y$ . $\newline$ Find an expression for $\frac{d^{2} y}{d x^{2}}$ in terms of $x$ and $y$ . $\newline$ $\frac{d^{2} y}{d x^{2}}=$

Full solution

More problems from Find the vertex of the transformed function

Related topics

We are given that dydx=x2−2y \frac{d y}{d x}=x^{2}-2 y dxdy​=x2−2y.\newlineFind an expression for d2ydx2 \frac{d^{2} y}{d x^{2}} dx2d2y​ in terms of x x x and y y y.\newlined2ydx2= \frac{d^{2} y}{d x^{2}}= dx2d2y​=

We are given that $\frac{d y}{d x}=x^{2}-2 y$ . $\newline$ Find an expression for $\frac{d^{2} y}{d x^{2}}$ in terms of $x$ and $y$ . $\newline$ $\frac{d^{2} y}{d x^{2}}=$