Full solution
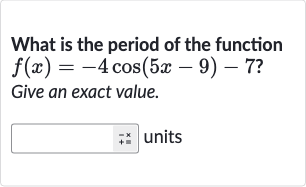
Q. What is the period of the function ?Give an exact value.units
- Identify standard form: Identify the standard form of the cosine function to determine the period.The standard form of a cosine function is , where:- is the amplitude,- affects the period of the function,- is the phase shift, and- is the vertical shift.In the given function , we can see that .
- Calculate period: Calculate the period of the function using the value of B.The period of a cosine function is given by the formula .For the given function, , so we calculate the period as follows:.
- Verify calculation: Verify that there are no mathematical errors in the calculation.The calculation of the period is straightforward and involves basic arithmetic. There are no mathematical errors in the calculation.
More problems from Domain and range of quadratic functions: equations
QuestionGet tutor help