Full solution
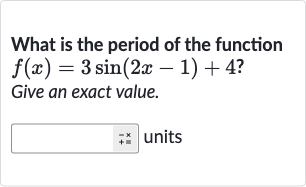
Q. What is the period of the function ?Give an exact value.units
- Determining the Period: The period of a sine function is determined by the coefficient of inside the sine function. The general form of a sine function is , where the period is given by .
- Finding the Coefficient of
x : For the functionf(x) = , the coefficient of3 3 2 2 1 1 4 4 x inside the sine function is2 2 2 2 b = .2 2 - Using the Period Formula: Calculate the period using the formula: Period =
2 π / b = 2 π / 2 2\pi/b = 2\pi/2 - Calculating the Period: Simplify the expression to find the period: Period =
π \pi
More problems from Domain and range of quadratic functions: equations
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help