Full solution
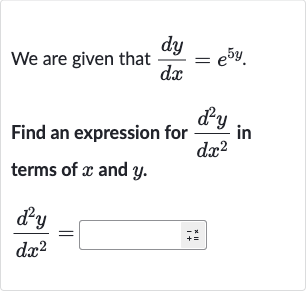
Q. We are given that .Find an expression for in terms of and .
- Given derivative: We are given the first derivative of with respect to as . To find the second derivative , we need to differentiate with respect to .
- Apply chain rule: Using the chain rule, we differentiate with respect to . Since is a function of , and is a function of , we apply the chain rule as follows: .
- Differentiate with respect to : Differentiate with respect to to get . So, .
- Substitute given derivative: Substitute the given into the expression. We get .
- Simplify the expression: Simplify the expression by combining the exponential terms. We have .
More problems from Find the vertex of the transformed function
QuestionGet tutor help