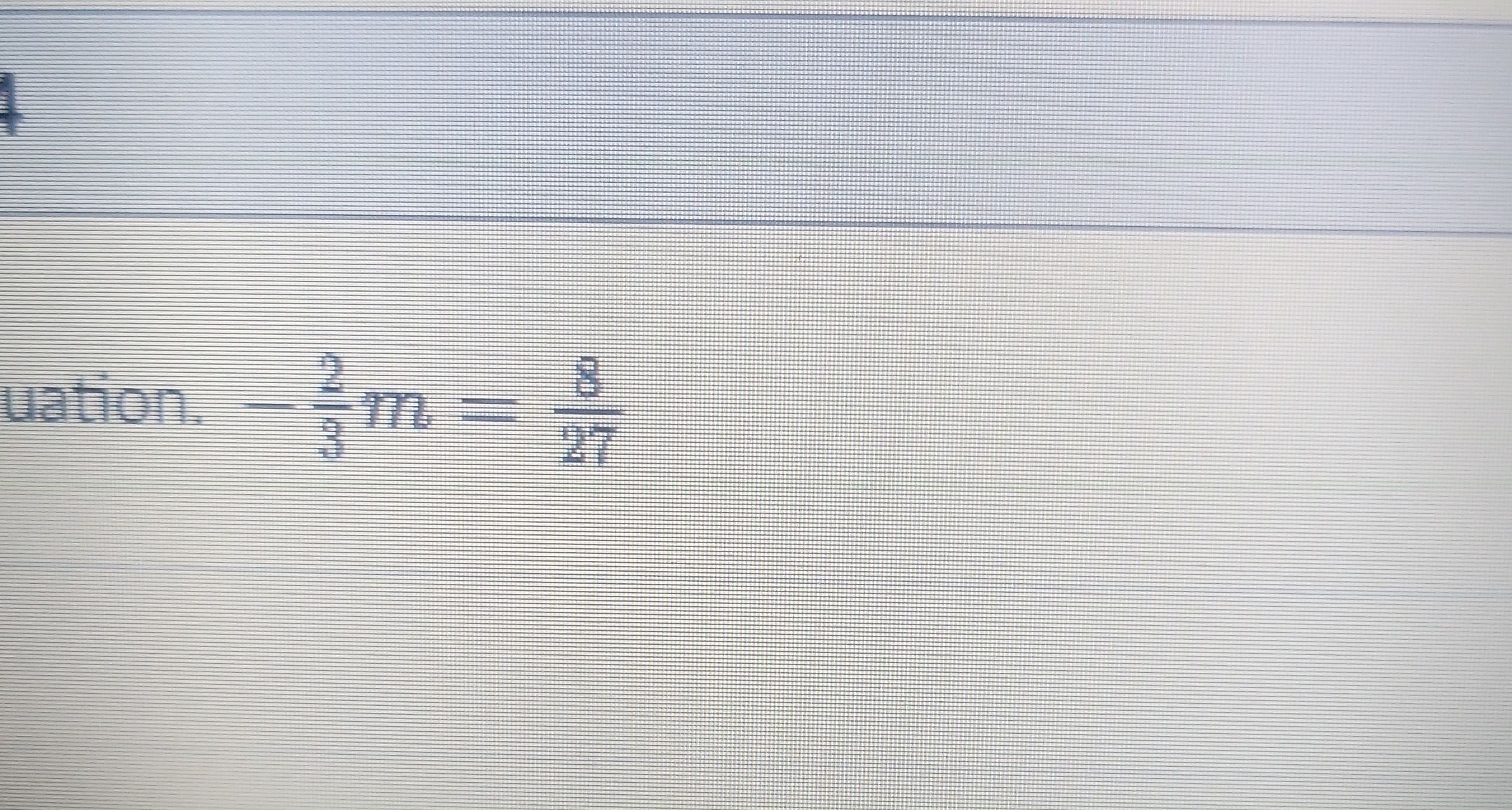AI tutor
Full solution
Q.
- Identify equation and goal: Identify the equation and the goal of solving for . We have the equation and we want to find the value of .
- Isolate m by multiplying: Isolate m by multiplying both sides of the equation by the reciprocal of , which is .Multiplying both sides by gives us .
- Simplify right side: Simplify the right side of the equation by multiplying the numerators and denominators..
- Reduce fraction: Reduce the fraction by finding the greatest common divisor (GCD) of and , which is . Divide both the numerator and the denominator by . .
- Check solution: Check the solution by substituting back into the original equation.. Simplify the left side: , which matches the right side.
More problems from Evaluate rational exponents
QuestionGet tutor help
QuestionGet tutor help