Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

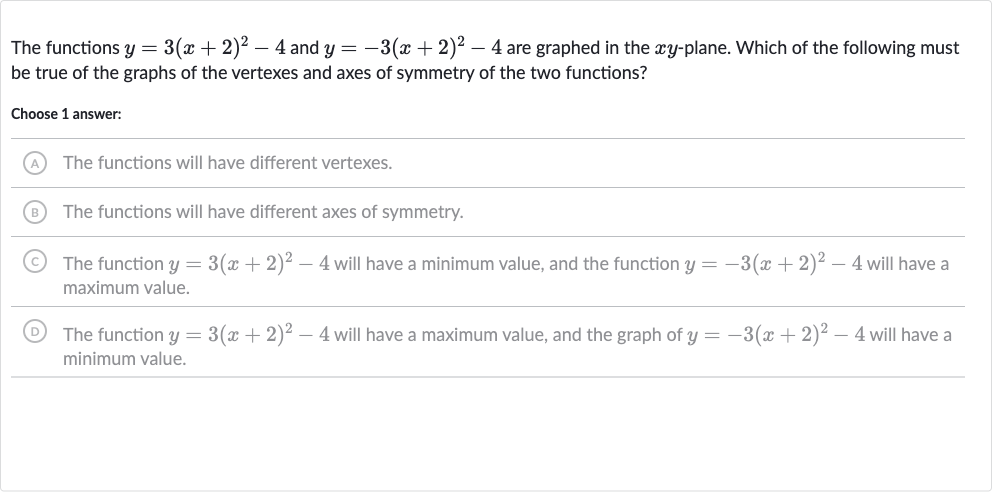
The functions y=3(x+2)^(2)-4 and y=-3(x+2)^(2)-4 are graphed in the xy-plane. Which of the following must be true of the graphs of the vertexes and axes of symmetry of the two functions? Choose 1 answer: (A) The functions will have different vertexes. (B) The functions will have different axes of symmetry. (C) The function y=3(x+2)^(2)-4 will have a minimum value, and the function y=-3(x+2)^(2)-4 will have a maximum value. (D) The function y=3(x+2)^(2)-4 will have a maximum value, and the graph of y=-3(x+2)^(2)-4 will have a minimum value.

The functions y=3(x+2)2−4 y=3(x+2)^{2}-4 and y=−3(x+2)2−4 y=-3(x+2)^{2}-4 are graphed in the xy x y -plane. Which of the following must be true of the graphs of the vertexes and axes of symmetry of the two functions?\newlineChoose 11 answer:\newline(A) The functions will have different vertexes.\newline(B) The functions will have different axes of symmetry.\newline(C) The function y=3(x+2)2−4 y=3(x+2)^{2}-4 will have a minimum value, and the function y=−3(x+2)2−4 y=-3(x+2)^{2}-4 will have a maximum value.\newline(D) The function y=3(x+2)2−4 y=3(x+2)^{2}-4 will have a maximum value, and the graph of y=−3(x+2)2−4 y=-3(x+2)^{2}-4 will have a minimum value.

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Precalculusright chevron icon
Solve trigonometric equationsright chevron icon

Full solution

Q. The functions y=3(x+2)2−4 y=3(x+2)^{2}-4 and y=−3(x+2)2−4 y=-3(x+2)^{2}-4 are graphed in the xy x y -plane. Which of the following must be true of the graphs of the vertexes and axes of symmetry of the two functions?\newlineChoose 11 answer:\newline(A) The functions will have different vertexes.\newline(B) The functions will have different axes of symmetry.\newline(C) The function y=3(x+2)2−4 y=3(x+2)^{2}-4 will have a minimum value, and the function y=−3(x+2)2−4 y=-3(x+2)^{2}-4 will have a maximum value.\newline(D) The function y=3(x+2)2−4 y=3(x+2)^{2}-4 will have a maximum value, and the graph of y=−3(x+2)2−4 y=-3(x+2)^{2}-4 will have a minimum value.
  1. Identify Form: Identify the form of the given functions.\newlineBoth functions are in the form of a transformed quadratic function y=a(x−h)2+ky=a(x-h)^2+k, where (h,k)(h, k) is the vertex of the parabola and x=hx=h is the axis of symmetry.
  2. Determine Vertex: Determine the vertex of each function.\newlineFor y=3(x+2)2−4y=3(x+2)^2-4, the vertex is (−2,−4)(-2, -4).\newlineFor y=−3(x+2)2−4y=-3(x+2)^2-4, the vertex is also (−2,−4)(-2, -4).\newlineSince both functions have the same hh and kk values in their vertex form, they share the same vertex.
  3. Axis of Symmetry: Determine the axis of symmetry for each function.\newlineThe axis of symmetry for both functions is x=−2x=-2, as it is derived from the hh value in the vertex (h,k)(h, k).
  4. Parabola Direction: Determine the direction of the parabolas.\newlineThe coefficient of the squared term aa determines the direction of the parabola. If aa is positive, the parabola opens upwards. If aa is negative, the parabola opens downwards.\newlineFor y=3(x+2)2−4y=3(x+2)^2-4, aa is positive, so the parabola opens upwards and has a minimum value.\newlineFor y=−3(x+2)2−4y=-3(x+2)^2-4, aa is negative, so the parabola opens downwards and has a maximum value.

More problems from Solve trigonometric equations

Question
Marco's class is painting a mural on the side of their school. The mural covers a 312 m 3 \frac{1}{2} \mathrm{~m} high, rectangular wall. It has an area of 28 m2 28 \mathrm{~m}^{2} .\newlineWhat is the length of the mural?\newlinem
Get tutor helpright-arrow

Posted 10 months ago

Question
A cup of hot coffee has been left to cool in a room with an ambient temperature of 21∘C 21^{\circ} \mathrm{C} .\newlineThe relationship between the elapsed time, m m , in minutes, since the coffee was left to cool, and the temperature of the coffee, T T , measured in ∘C { }^{\circ} \mathrm{C} , is modeled by the following function.\newlineT(m)=21+74⋅10−0.03m T(m)=21+74 \cdot 10^{-0.03 m} \newlineWhat will the temperature of the coffee be after 1010 minutes?\newlineRound your answer, if necessary, to the nearest hundredth.\newline∘C { }^{\circ} C
Get tutor helpright-arrow

Posted 10 months ago

Question
Takumi plants a tree in his backyard and studies how the number of branches grows over time.\newlineHe predicts that the relationship between N N , the number of branches on the tree, and t t , the elapsed time, in years, since the tree was planted can be modeled by the following equation.\newlineN=5⋅100.3t N=5 \cdot 10^{0.3 t} \newlineAccording to Takumi's model, in how many years will the tree have 100100 branches?\newlineGive an exact answer expressed as a base−10-10 logarithm.\newlineyears
Get tutor helpright-arrow

Posted 10 months ago

Question
Let g(x)=2x g(x)=2^{x} .\newlineCan we use the mean value theorem to say the equation g′(x)=16 g^{\prime}(x)=16 has a solution where 3<x<5 3<x<5 ?\newlineChoose 11 answer:\newline(A) No, since the function is not differentiable on that interval.\newline(B) No, since the average rate of change of g g over the interval 3≤x≤5 3 \leq x \leq 5 isn't equal to 16‾ 1 \overline{6} .\newline(C) Yes, both conditions for using the mean value theorem have been met.
Get tutor helpright-arrow

Posted 9 months ago

Question
ddx(3x2−1x−2)= \frac{d}{d x}\left(\frac{3 x^{2}-1}{x-2}\right)=
Get tutor helpright-arrow

Posted 9 months ago

Question
dydt=2t+3 and y(1)=6 \frac{d y}{d t}=2 t+3 \text { and } y(1)=6 \newlineWhat is t t when y=0 y=0 ?\newlineChoose all answers that apply:\newline(A) t=−1 t=-1 \newline(B) t=−3 t=-3 \newline(C) t=0 t=0 \newline(D) t=−4 t=-4 \newline(E) t=1 t=1 \newline(F) t=−2 t=-2
Get tutor helpright-arrow

Posted 9 months ago

Question
ddx(x34)= \frac{d}{d x}\left(x^{\frac{3}{4}}\right)=
Get tutor helpright-arrow

Posted 9 months ago

Question
y=x13 y=x^{13} \newlinedydx= \frac{d y}{d x}=
Get tutor helpright-arrow

Posted 9 months ago

Question
y=x10 y=x^{10} \newlinedydx= \frac{d y}{d x}=
Get tutor helpright-arrow

Posted 9 months ago

Question
ddx[x6]= \frac{d}{d x}\left[x^{6}\right]=
Get tutor helpright-arrow

Posted 9 months ago

View all (6)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant