AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
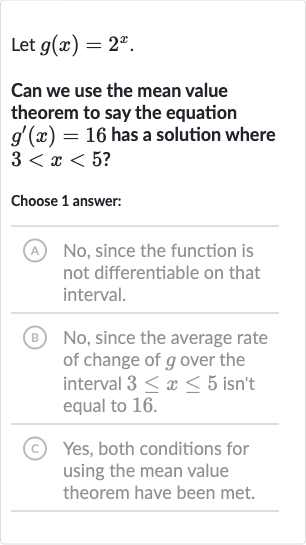
Let .Can we use the mean value theorem to say the equation has a solution where \( 3
Full solution
Q. Let .Can we use the mean value theorem to say the equation has a solution where ?Choose answer:(A) No, since the function is not differentiable on that interval.(B) No, since the average rate of change of over the interval isn't equal to .(C) Yes, both conditions for using the mean value theorem have been met.
- Recall Mean Value Theorem: First, let's recall the Mean Value Theorem (MVT). The MVT states that if a function is continuous on a closed interval and differentiable on the open interval , then there exists at least one in such that . We need to check if satisfies these conditions on the interval .
- Check Conditions: The function is a continuous and differentiable function for all real numbers, including the interval . Therefore, the first condition of the MVT is satisfied.
- Calculate Average Rate of Change: Next, we calculate the average rate of change of over the interval . This is given by .
- Compare Average Rate of Change: Now we compare the average rate of change, which is , to the value given in the problem, which is . Since does not equal , the average rate of change of over the interval is not equal to .
- Apply Mean Value Theorem: Since the average rate of change of on is not equal to , we cannot guarantee that there is a point in where equals , based on the Mean Value Theorem. Therefore, the correct answer is (B) No, since the average rate of change of over the interval isn't equal to .
