The derivative of a function $g$ is given by $\newline$ $g^{\prime}(x)=x^{3}-2 x^{2}-3 x+4 .$ $\newline$ On which intervals is the graph of $g$ increasing? $\newline$ Use a graphing calculator. $\newline$ Choose $1$ answer: $\newline$ (A) $x \leq-1.562$ and $\newline$ $1 \leq x \leq 2.562$ $\newline$ (B) $-1.562 \leq x \leq 1$ and $x \geq 2.562$ $\newline$ (C) $x \leq-0.535$ and $x \geq 1.869$ $\newline$ (D) $-0.535 \leq x \leq 1.869$ $\newline$ (E) All real numbers

Full solution

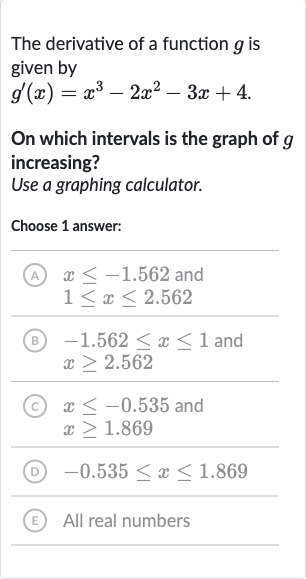
Q. The derivative of a function

g

is given by

\newline

g^{\prime}(x)=x^{3}-2 x^{2}-3 x+4 .

\newline

On which intervals is the graph of

g

increasing?

\newline

Use a graphing calculator.

\newline

Choose

1

answer:

\newline

(A)

x \leq-1.562

and

\newline

1 \leq x \leq 2.562

\newline

(B)

-1.562 \leq x \leq 1

and

x \geq 2.562

\newline

(C)

x \leq-0.535

and

x \geq 1.869

\newline

(D)

-0.535 \leq x \leq 1.869

\newline

(E) All real numbers

Find Critical Points: Find the critical points of $g'(x)$ by setting the derivative equal to zero and solving for $x$ . $g'(x) = x^3 - 2x^2 - 3x + 4 = 0$
Use Graphing Calculator: Use a graphing calculator to find the roots of the equation. $\newline$ Roots are approximately $x = -0.535$ , $x = 1$ , and $x = 1.869$ .
Determine Sign of $g'(x)$ : Determine the sign of $g'(x)$ on the intervals determined by the critical points. $\newline$ Test points: $x = -1$ , $x = 0.5$ , $x = 1.5$ , and $x = 2$ .
Plug in Test Points: Plug test points into $g'(x)$ to check if the derivative is positive (increasing) or negative (decreasing). $\newline$ For $x = -1$ : $g'(-1) > 0$ (increasing) $\newline$ For $x = 0.5$ : $g'(0.5) < 0$ (decreasing) $\newline$ For $x = 1.5$ : $g'(1.5) > 0$ (increasing) $\newline$ For $x = 2$ : $g'(2) < 0$ (decreasing)
Graph of g is Increasing: Based on the sign of $g'(x)$ , the graph of $g$ is increasing on the intervals where $g'(x) > 0$ . $\newline$ Increasing intervals: $(-\infty, -0.535)$ and $(1, 1.869)$ .