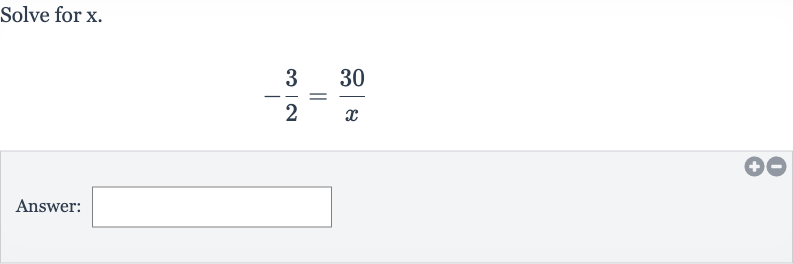AI tutor
Full solution
Q. Solve for .Answer:
- Identify Equation: Identify the equation to solve for . We have the equation . We need to find the value of that satisfies this equation.
- Cross-Multiply: Cross-multiply to solve for . Cross-multiplication gives us .
- Simplify Equation: Simplify the right side of the equation. equals , so the equation becomes .
- Divide for x: Divide both sides by to solve for . Dividing both sides by gives us .
- Calculate : Calculate the value of ., so .
More problems from Evaluate rational exponents
QuestionGet tutor help
QuestionGet tutor help