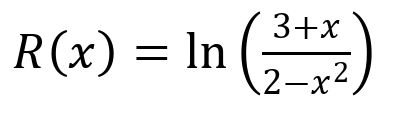R(x)=ln((3+x)/(2-x^(2)))

Question

Bytelearn · Accepted Answer

Identify Function: Identify the function that we need to differentiate. R(x) = ln((3+x)/(2-x^2)) We need to find R'(x), the derivative of R with respect to x.Apply Chain Rule: Apply the chain rule for differentiation to the natural logarithm function. The derivative of ln(u) with respect to x is 1/u * du/dx, where u is a function of x. In this case, u = (3+x)/(2-x^2).Differentiate Numerator and Denominator: Differentiate the numerator and the denominator of u separately. The derivative of the numerator, 3+x, with respect to x is 1. The derivative of the denominator, 2-x^2, with respect to x is -2x.Apply Quotient Rule: Apply the quotient rule to differentiate u = (3+x)/(2-x^2). The quotient rule is given by (v * du/dx - u * dv/dx) / v^2, where u is the numerator and v is the denominator.Perform Quotient Rule Calculations: Perform the calculations for the quotient rule. Let u = 3+x and v = 2-x^2. du/dx = 1 and dv/dx = -2x. Now, apply the quotient rule: (v * du/dx - u * dv/dx) / v^2 = ((2-x^2) * 1 - (3+x) * (-2x)) / (2-x^2)^2.Simplify Expression: Simplify the expression obtained from the quotient rule. ((2-x^2) * 1 - (3+x) * (-2x)) / (2-x^2)^2 = (2 - x^2 + 2x(3+x)) / (2-x^2)^2.Expand and Combine Terms: Expand and combine like terms in the numerator. 2 - x^2 + 2x(3+x) = 2 - x^2 + 6x + 2x^2. Combine like terms: 2 + 6x + x^2.Write Final Derivative Expression: Write the final expression for the derivative of R(x). R'(x) = (2 + 6x + x^2) / (2-x^2)^2. This is the derivative of the function R(x) with respect to x.

$R(x)=\ln \left(\frac{3+x}{2-x^{2}}\right)$

Full solution

More problems from Evaluate rational exponents

Related topics

R(x)=ln⁡(3+x2−x2) R(x)=\ln \left(\frac{3+x}{2-x^{2}}\right) R(x)=ln(2−x23+x​)

$R(x)=\ln \left(\frac{3+x}{2-x^{2}}\right)$