Full solution
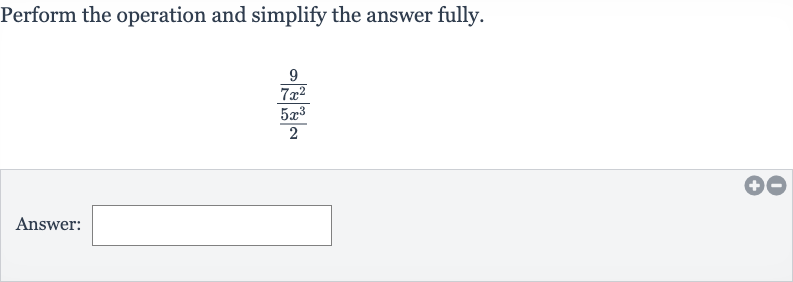
Q. Perform the operation and simplify the answer fully.Answer:
- Identify Given Expression: Identify the given expression and rewrite it as a single division problem by multiplying by the reciprocal of the divisor.The expression can be rewritten as .
- Simplify by Multiplying: Simplify the expression by multiplying the numerators and denominators..
- Combine X Terms: Combine the terms in the denominator by adding the exponents.Since , we have .
- Check for Further Simplification: Check if the expression can be simplified further. The numbers and do not have common factors other than , and the terms are already combined, so the expression is fully simplified.
More problems from Operations with rational exponents
QuestionGet tutor help
QuestionGet tutor help