Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

log_(5)25=

log⁡525= \log _{5} 25=

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Grade 8right chevron icon
Relationship between squares and square rootsright chevron icon

Full solution

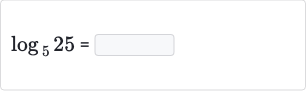
Q. log⁡525= \log _{5} 25=
  1. Find logarithm value: We need to find the value of log⁡525\log_{5}25. The logarithm log⁡ba\log_{b}a answers the question: ＂To what power must the base bb be raised, to produce the number aa?＂
  2. Rewrite expression using property: Since 2525 is a perfect square and can be expressed as 525^2, we can rewrite the expression using the property of logarithms that states log⁡b(bx)=x\log_{b}(b^x) = x.
  3. Substitute 2525 in expression: Substitute 2525 with 55^22 in the logarithmic expression: \log_{55}(55^22).
  4. Apply logarithm property: Apply the logarithm property to simplify the expression: log⁡5(52)=2\log_{5}(5^2) = 2.
  5. Final logarithm value: We have found the value of the logarithm: log⁡525=2\log_{5}25 = 2.

More problems from Relationship between squares and square roots

Question
Solve the following equation for y y .\newliney+6=2y2+72y=□ \begin{array}{l} y+6=\sqrt{2 y^{2}+72} \\ y=\square \end{array}
Get tutor helpright-arrow

Posted 3 months ago

Question
z=12.1+29i z=12.1+29 i \newlineWhat is the real part of z z ?\newlineWhat is the imaginary part of z z ?
Get tutor helpright-arrow

Posted 3 months ago

Question
z=−400i−44.5 z=-400 i-44.5 \newlineWhat is the real part of z z ?\newlineWhat is the imaginary part of z z ?
Get tutor helpright-arrow

Posted 3 months ago

Question
z=−91+14.6i z=-91+14.6 i \newlineWhat is the real part of z z ?\newlineWhat is the imaginary part of z z ?
Get tutor helpright-arrow

Posted 3 months ago

Question
z=44i+23.3 z=44 i+23.3 \newlineWhat is the real part of z z ?\newlineWhat is the imaginary part of z z ?
Get tutor helpright-arrow

Posted 3 months ago

Question
log⁡168= \log _{16} 8=
Get tutor helpright-arrow

Posted 3 months ago

Question
log⁡497= \log _{49} 7=
Get tutor helpright-arrow

Posted 3 months ago

Question
log⁡327= \log _{3} 27=
Get tutor helpright-arrow

Posted 3 months ago

Question
log⁡749= \log _{7} 49=
Get tutor helpright-arrow

Posted 3 months ago

Question
(88) How many liters of pure water should be added to 150150 liters of a 35%35\% salt water saline solution to make it a 25%25\% salt water saline solution? Let =\quad = \quad and Let 11=\quad 11 =\quad
Get tutor helpright-arrow

Posted 4 hours ago

View all (19)