Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

log_(49)7=

$\log _{49} 7=$

View step-by-step help

View step-by-step help

Relationship between squares and square roots

Full solution

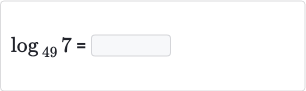
Q.

\log _{49} 7=

Problem: We need to find the value of the logarithm $\log_{49}7$ . The base of the logarithm is $49$ , and the number we are taking the log of is $7$ . We are looking for the exponent that we need to raise $49$ to in order to get $7$ .
Definition of Logarithm: Recall the definition of a logarithm: if $a^x = b$ , then $\log_a(b) = x$ . We need to find an exponent $x$ such that $49^x = 7$ .
Rewriting the Base: We know that $49$ is a perfect square, specifically $49 = 7^2$ . Therefore, we can rewrite $49^x$ as $(7^2)^x$ .
Simplifying the Exponent: Using the property of exponents that $(a^b)^c = a^{(b*c)}$ , we can simplify $(7^2)^x$ to $7^{(2*x)}$ .
Setting up the Equation: We want $7^{2x}$ to equal $7$ , which is the same as $7^1$ . Therefore, we need $2x$ to equal $1$ .
Solving for x: To find x, we divide both sides of the equation $2x = 1$ by $2$ , which gives us $x = \frac{1}{2}$ .
Final Result: Therefore, $\log_{49}7$ is equal to $\frac{1}{2}$ because raising $49$ (which is $7^2$ ) to the power of $\frac{1}{2}$ gives us $7$ .

More problems from Relationship between squares and square roots

Question

Solve the following equation for

y

\newline

\begin{array}{l} y+6=\sqrt{2 y^{2}+72} \\ y=\square \end{array}

Posted 3 months ago

Question

z=12.1+29 i

\newline

What is the real part of

z

\newline

What is the imaginary part of

z

Posted 3 months ago

Question

z=-400 i-44.5

\newline

What is the real part of

z

\newline

What is the imaginary part of

z

Posted 3 months ago

Question

z=-91+14.6 i

\newline

What is the real part of

z

\newline

What is the imaginary part of

z

Posted 3 months ago

Question

z=44 i+23.3

\newline

What is the real part of

z

\newline

What is the imaginary part of

z

Posted 3 months ago

Question

\log _{16} 8=

Posted 3 months ago

Question

\log _{5} 25=

Posted 3 months ago

Question

\log _{3} 27=

Posted 3 months ago

Question

\log _{7} 49=

Posted 3 months ago

Question

Plot the vertex. Then plot another point on the parabola. If you make a mistake, you can erase your parabola by selecting the second point and placing it on top of the first.

\newline

\newline

Posted 7 hours ago