Full solution
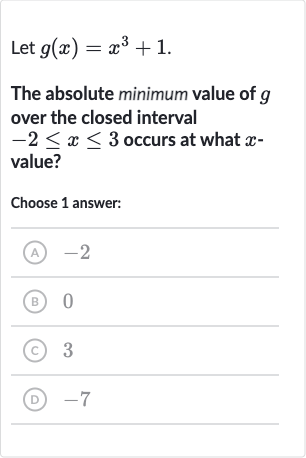
Q. Let .The absolute minimum value of over the closed interval occurs at what value?Choose answer:(A) (B) (C) (D)
- Identify Critical Points: To find the absolute minimum value of the function over the closed interval , we need to evaluate the function at the critical points and the endpoints of the interval.
- Evaluate Function at Critical Points: First, we find the critical points by taking the derivative of and setting it equal to zero.Setting gives us , which implies .
- Evaluate Function at Endpoints: Now we evaluate the function at the critical point and at the endpoints of the interval, and .
- Compare Values and Determine Minimum: Comparing the values of at , , and , we see that the smallest value is . Therefore, the absolute minimum value of over the interval occurs at .
More problems from Solve quadratic inequalities
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help