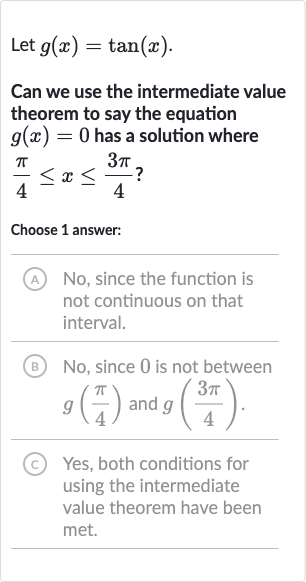
Let $g(x)=\tan (x)$ . $\newline$ Can we use the intermediate value theorem to say the equation $g(x)=0$ has a solution where $\frac{\pi}{4} \leq x \leq \frac{3 \pi}{4}$ ? $\newline$ Choose $1$ answer: $\newline$ (A) No, since the function is not continuous on that interval. $\newline$ (B) No, since $0$ is not between $g\left(\frac{\pi}{4}\right)$ and $g\left(\frac{3 \pi}{4}\right)$ . $\newline$ (C) Yes, both conditions for using the intermediate value theorem have been met.

View step-by-step help

Home

Math Problems

Algebra 2

Solve quadratic inequalities

Full solution

Q. Let

g(x)=\tan (x)

\newline

Can we use the intermediate value theorem to say the equation

g(x)=0

has a solution where

\frac{\pi}{4} \leq x \leq \frac{3 \pi}{4}

\newline

Choose

1

answer:

\newline

(A) No, since the function is not continuous on that interval.

\newline

(B) No, since

0

is not between

g\left(\frac{\pi}{4}\right)

and

g\left(\frac{3 \pi}{4}\right)

\newline

Understand IVT: Understand the Intermediate Value Theorem (IVT). The IVT states that if a function $f$ is continuous on a closed interval $[a, b]$ and $k$ is any number between $f(a)$ and $f(b)$ , then there is at least one number $c$ in the interval $[a, b]$ such that $f(c) = k$ .
Check Continuity: Check if $g(x) = \tan(x)$ is continuous on the interval $[\frac{\pi}{4}, \frac{3\pi}{4}]$ . The function $\tan(x)$ is continuous wherever it is defined. However, $\tan(x)$ has vertical asymptotes at odd multiples of $\frac{\pi}{2}$ , which means it is not continuous at these points. Since $\frac{3\pi}{4}$ is less than $\frac{\pi}{2}$ , there are no vertical asymptotes within the interval $[\frac{\pi}{4}, \frac{3\pi}{4}]$ , and thus $g(x)$ is continuous on this interval.
Evaluate Endpoints: Evaluate $g(x)$ at the endpoints of the interval. $\newline$ Calculate $g(\frac{\pi}{4}) = \tan(\frac{\pi}{4})$ and $g(\frac{3\pi}{4}) = \tan(\frac{3\pi}{4})$ . $\newline$ $g(\frac{\pi}{4}) = \tan(\frac{\pi}{4}) = 1$ $\newline$ $g(\frac{3\pi}{4}) = \tan(\frac{3\pi}{4}) = -1$
Check Value Range: Check if $0$ is between $g(\frac{\pi}{4})$ and $g(\frac{3\pi}{4})$ . $\newline$ Since $g(\frac{\pi}{4}) = 1$ and $g(\frac{3\pi}{4}) = -1$ , the value $0$ is indeed between these two values.
Apply IVT: Apply the Intermediate Value Theorem. $\newline$ Since $g(x)$ is continuous on the interval $[\frac{\pi}{4}, \frac{3\pi}{4}]$ and $0$ is between $g(\frac{\pi}{4})$ and $g(\frac{3\pi}{4})$ , the IVT confirms that there is at least one value $c$ in the interval $[\frac{\pi}{4}, \frac{3\pi}{4}]$ such that $g(c) = 0$ .