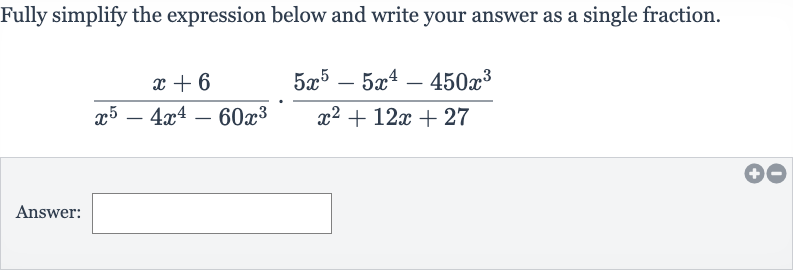
Fully simplify the expression below and write your answer as a single fraction. (x+6)/(x^(5)-4x^(4)-60x^(3))*(5x^(5)-5x^(4)-450x^(3))/(x^(2)+12 x+27) Answer:

Question

Fully simplify the expression below and write your answer as a single fraction.  (x+6)/(x^(5)-4x^(4)-60x^(3))*(5x^(5)-5x^(4)-450x^(3))/(x^(2)+12 x+27) Answer:

Bytelearn · Accepted Answer

Factor Denominator First Fraction: First, factor the polynomials in the numerator and the denominator where possible. Starting with the denominator of the first fraction: $x^5 - 4x^4 - 60x^3$. Factor out the common term $x^3$: $x^3(x^2 - 4x - 60)$. Now factor the quadratic: $x^2 - 4x - 60 = (x - 10)(x + 6)$. So the denominator becomes: $x^3(x - 10)(x + 6)$.Factor Numerator Second Fraction: Next, factor the numerator of the second fraction: $5x^5 - 5x^4 - 450x^3$. Factor out the common term $5x^3$: $5x^3(x^2 - x - 90)$. Now factor the quadratic: $x^2 - x - 90 = (x - 10)(x + 9)$. So the numerator becomes: $5x^3(x - 10)(x + 9)$.Factor Denominator Second Fraction: Now, factor the denominator of the second fraction: $x^2 + 12x + 27$. This is a simple quadratic that factors to: $(x + 3)(x + 9)$.Rewrite Expression with Factored Terms: We can now rewrite the entire expression with the factored terms: $$\frac{(x+6)}{x^3(x - 10)(x + 6)} \cdot \frac{5x^3(x - 10)(x + 9)}{(x + 3)(x + 9)}$$Cancel Common Terms: Next, cancel out the common terms in the numerator and the denominator. The $x + 6$ terms cancel each other, as do the $x - 10$ terms and the $x + 9$ terms. We are left with: $$\frac{5x^3}{x^3(x + 3)}$$Cancel Common x^3 Term: Now, cancel out the common $x^3$ term: $$\frac{5}{x + 3}$$Final Simplified Answer: The expression is now fully simplified, and there are no more common terms to cancel. The final answer is: $$\frac{5}{x + 3}$$

Fully simplify the expression below and write your answer as a single fraction. $\newline$ $\frac{x+6}{x^{5}-4 x^{4}-60 x^{3}} \cdot \frac{5 x^{5}-5 x^{4}-450 x^{3}}{x^{2}+12 x+27}$ $\newline$ Answer:

Full solution

More problems from Operations with rational exponents

Related topics

Fully simplify the expression below and write your answer as a single fraction.\newlinex+6x5−4x4−60x3⋅5x5−5x4−450x3x2+12x+27 \frac{x+6}{x^{5}-4 x^{4}-60 x^{3}} \cdot \frac{5 x^{5}-5 x^{4}-450 x^{3}}{x^{2}+12 x+27} x5−4x4−60x3x+6​⋅x2+12x+275x5−5x4−450x3​\newlineAnswer:

Fully simplify the expression below and write your answer as a single fraction. $\newline$ $\frac{x+6}{x^{5}-4 x^{4}-60 x^{3}} \cdot \frac{5 x^{5}-5 x^{4}-450 x^{3}}{x^{2}+12 x+27}$ $\newline$ Answer: