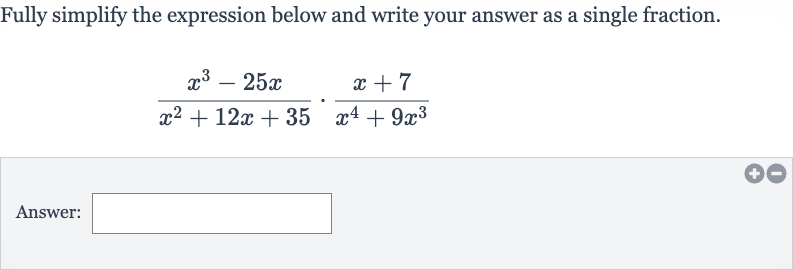
Fully simplify the expression below and write your answer as a single fraction. (x^(3)-25 x)/(x^(2)+12 x+35)*(x+7)/(x^(4)+9x^(3)) Answer:

Question

Fully simplify the expression below and write your answer as a single fraction.  (x^(3)-25 x)/(x^(2)+12 x+35)*(x+7)/(x^(4)+9x^(3)) Answer:

Bytelearn · Accepted Answer

Identify factors: Identify the factors in the numerator and denominator that can be factored further. The numerator x^3 - 25x can be factored out by taking x common, resulting in x(x^2 - 25). The denominator x^2 + 12x + 35 can be factored into (x + 5)(x + 7). The second fraction's numerator is already simplified as x + 7. The second fraction's denominator x^4 + 9x^3 can be factored by taking x^3 common, resulting in x^3(x + 9).Factor numerator and denominator: Factor the expression x^2 - 25 in the numerator, which is a difference of squares. x^2 - 25 = (x + 5)(x - 5). Now the expression becomes x(x + 5)(x - 5) / (x + 5)(x + 7) * (x + 7) / x^3(x + 9).Factor expression: Cancel out the common factors in the numerator and the denominator. The (x + 5) in the numerator cancels with the (x + 5) in the denominator. The (x + 7) in the numerator cancels with the (x + 7) in the denominator. We are left with x(x - 5) / x^3(x + 9).Cancel common factors: Simplify the expression by canceling the common x terms. One x in the numerator cancels with one x in the denominator, leaving us with (x - 5) / x^2(x + 9).Simplify expression: Write the final simplified expression. The fully simplified expression is (x - 5) / (x^2(x + 9)).

Fully simplify the expression below and write your answer as a single fraction. $\newline$ $\frac{x^{3}-25 x}{x^{2}+12 x+35} \cdot \frac{x+7}{x^{4}+9 x^{3}}$ $\newline$ Answer:

Full solution

More problems from Operations with rational exponents

Related topics

Fully simplify the expression below and write your answer as a single fraction.\newlinex3−25xx2+12x+35⋅x+7x4+9x3 \frac{x^{3}-25 x}{x^{2}+12 x+35} \cdot \frac{x+7}{x^{4}+9 x^{3}} x2+12x+35x3−25x​⋅x4+9x3x+7​\newlineAnswer:

Fully simplify the expression below and write your answer as a single fraction. $\newline$ $\frac{x^{3}-25 x}{x^{2}+12 x+35} \cdot \frac{x+7}{x^{4}+9 x^{3}}$ $\newline$ Answer: