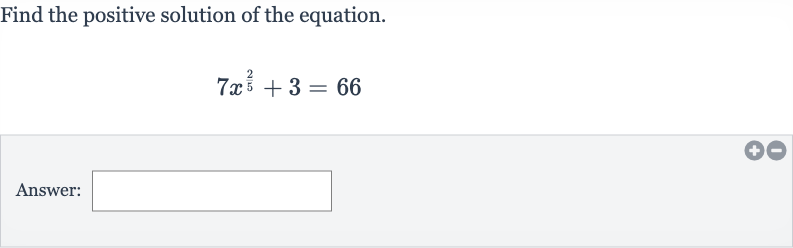
Find the positive solution of the equation. 7x^((2)/(5))+3=66 Answer:

Question

Find the positive solution of the equation.  7x^((2)/(5))+3=66 Answer:

Bytelearn · Accepted Answer

Isolate variable term: First, we need to isolate the term containing the variable $x$. To do this, we subtract 3 from both sides of the equation. $7x^{\frac{2}{5}} + 3 - 3 = 66 - 3$Simplify the equation: Now, we simplify the equation by performing the subtraction. $7x^{\frac{2}{5}} = 63$Divide by 7: Next, we divide both sides of the equation by 7 to solve for $x^{\frac{2}{5}}$. $\frac{7x^{\frac{2}{5}}}{7} = \frac{63}{7}$Remove fractional exponent: We calculate the division on both sides to find the value of $x^{\frac{2}{5}}$. $x^{\frac{2}{5}} = 9$Raise to reciprocal: To solve for $x$, we need to get rid of the fractional exponent. We do this by raising both sides of the equation to the reciprocal of $\frac{2}{5}$, which is $\frac{5}{2}$. $(x^{\frac{2}{5}})^{\frac{5}{2}} = 9^{\frac{5}{2}}$Calculate 9^5/2: When we raise a power to a power, we multiply the exponents. In this case, $\frac{2}{5} \times \frac{5}{2} = 1$, so we are left with $x$ on the left side of the equation. $x = 9^{\frac{5}{2}}$Rewrite as 3^5: Now we need to calculate $9^{\frac{5}{2}}$. Since $9 = 3^2$, we can rewrite $9^{\frac{5}{2}}$ as $(3^2)^{\frac{5}{2}}$. $x = (3^2)^{\frac{5}{2}}$Calculate x: We apply the power to a power rule again, multiplying the exponents $2 \times \frac{5}{2} = 5$. $x = 3^5$Finally, we calculate $3^5$ to find the value of $x$. $x = 3 \times 3 \times 3 \times 3 \times 3$ $x = 243$

Find the positive solution of the equation. $\newline$ $7 x^{\frac{2}{5}}+3=66$ $\newline$ Answer:

Full solution

More problems from Operations with rational exponents

Related topics

Find the positive solution of the equation.\newline7x25+3=66 7 x^{\frac{2}{5}}+3=66 7x52​+3=66\newlineAnswer:

Find the positive solution of the equation. $\newline$ $7 x^{\frac{2}{5}}+3=66$ $\newline$ Answer: