Full solution
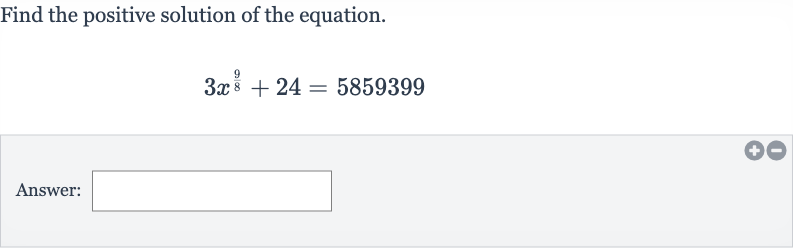
Q. Find the positive solution of the equation.Answer:
- Isolate variable term: Isolate the term with the variable.Subtract from both sides of the equation to isolate the term with the variable .
- Divide by : Divide both sides by to solve for .
- Recognize power of : Recognize that is a power of .
- Set equal and solve: Set the expression equal to and solve for .Since , we can now find by taking both sides to the power of .
- Calculate power of : Calculate .
More problems from Operations with rational exponents
QuestionGet tutor help
QuestionGet tutor help