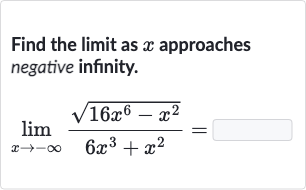Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Find the limit as x approaches negative infinity. lim_(x rarr-oo)(sqrt(16x^(6)-x^(2)))/(6x^(3)+x^(2))=

Find the limit as x x approaches negative infinity.\newlinelim⁡x→−∞16x6−x26x3+x2= \lim _{x \rightarrow-\infty} \frac{\sqrt{16 x^{6}-x^{2}}}{6 x^{3}+x^{2}}=

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 1right chevron icon
Compare linear and exponential growthright chevron icon

Full solution

Q. Find the limit as x x approaches negative infinity.\newlinelim⁡x→−∞16x6−x26x3+x2= \lim _{x \rightarrow-\infty} \frac{\sqrt{16 x^{6}-x^{2}}}{6 x^{3}+x^{2}}=
  1. Identify highest power of x: Identify the highest power of xx in the numerator and the denominator.\newlineThe highest power of xx in the numerator is x6x^6 (from the term 16x616x^6), and the highest power of xx in the denominator is x3x^3 (from the term 6x36x^3).
  2. Divide by highest power: Divide every term by x3x^3, the highest power of xx in the denominator.\newlineWe get (16x6x3−x2x3)/(6x3x3+x2x3)=(16x3−1x)/(6+1x)(\sqrt{\frac{16x^6}{x^3} - \frac{x^2}{x^3}})/(\frac{6x^3}{x^3} + \frac{x^2}{x^3}) = (\sqrt{16x^3 - \frac{1}{x}})/(6 + \frac{1}{x}).
  3. Simplify expression inside square root: Simplify the expression inside the square root.\newlineSince xx is approaching negative infinity, 1x\frac{1}{x} approaches 00. Therefore, the term −1x-\frac{1}{x} inside the square root becomes negligible, and we can approximate the expression as 16x36+1x\frac{\sqrt{16x^3}}{6 + \frac{1}{x}}.
  4. Simplify square root: Simplify the square root.\newlineThe square root of 16x316x^3 is 4x324x^{\frac{3}{2}}. So the expression simplifies to 4x326+1x\frac{4x^{\frac{3}{2}}}{6 + \frac{1}{x}}.
  5. Approach negative infinity: As xx approaches negative infinity, 1x\frac{1}{x} approaches 00. The term 1x\frac{1}{x} in the denominator becomes negligible, so the expression simplifies to 4x(3/2)6\frac{4x^{(3/2)}}{6}.
  6. Further simplify expression: Simplify the expression further.\newlineSince x(3/2)x^{(3/2)} is the same as (x3)(1/2)(x^3)^{(1/2)}, we can rewrite the expression as 46x(3/2)\frac{4}{6x^{(3/2)}}.

More problems from Compare linear and exponential growth

Question
Jill bought a used motorcycle from a seller online for $1,200. The seller will charge her $5 a day to store the motorcycle at his house until she is able to pick it up. You can use a function to describe the total amount of money Jill will owe the seller if she waits xx days to pick up the motorcycle.\newlineWrite an equation for the function. If it is linear, write it in the form g(x)=mx+bg(x) = mx + b. If it is exponential, write it in the form g(x)=a(b)xg(x) = a(b)^x.\newlineg(x)=‾g(x) = \underline{\hspace{3em}}
Get tutor helpright-arrow

Posted 5 months ago

Question
How does f(t)=−2t−7f(t)=-2t-7 change over the interval from t=−7t=-7 to t=−6t=-6?\newlineChoices:\newline[f(t) decreases by 2]\left[\text{f(t) decreases by } 2\right]\newline[f(t) increases by 2]\left[\text{f(t) increases by } 2\right]\newline[f(t) increases by 200%]\left[\text{f(t) increases by } 200\%\right]\newline[f(t) decreases by a factor of 2]\left[\text{f(t) decreases by a factor of } 2\right]
Get tutor helpright-arrow

Posted 5 months ago

Question
How does g(t)=9tg(t)=9^t change over the interval from t=1t=1 to t=3t=3?\newlineChoices: \newline[g(t) decreases by a factor of 81]\left[\text{g(t) decreases by a factor of } 81\right]\newline[g(t) increases by a factor of 81]\left[\text{g(t) increases by a factor of } 81\right]\newline[g(t) increases by 18%]\left[\text{g(t) increases by } 18\%\right]\newline[g(t) decreases by 9%]\left[\text{g(t) decreases by } 9\%\right]
Get tutor helpright-arrow

Posted 5 months ago

Question
Both of these functions grow as xx gets larger and larger. Which function eventually exceeds the other?\newline Choices:\newline(A)f(x)=8x+3.3(A)f(x) = 8x + 3.3\newline(B)g(x)=3.3x−5(B)g(x) = 3.3^x - 5
Get tutor helpright-arrow

Posted 6 months ago

Question
Both of these functions grow as x x gets larger and larger. Which function eventually exceeds the other?\newlineChoices:\newline(A) f(x)=2x+9(A) \ f(x) = 2x + 9 \newline(B) g(x)=2x(B)\ g(x) = 2^x
Get tutor helpright-arrow

Posted 6 months ago

Question
The functions f(x) f(x) and g(x) g(x) are differentiable. \newlineThe function h(x) h(x) is defined as: h(x)=g(x)f(x) h(x)=\frac{g(x)}{f(x)} \newlineIf f(8)=1 f(8)=1 , f′(8)=−6 f'(8)=-6 , g(8)=8 g(8)=8 , and g′(8)=−10 g'(8)=-10 , what is h′(8) h'(8) ? \newlineSimplify any fractions. \newlineh′(8)= h'(8)= ______
Get tutor helpright-arrow

Posted 6 months ago

Question
The functions p(x) p(x) and q(x) q(x) are differentiable. \newlineThe function r(x) r(x) is defined as: r(x)=p(x)q(x) r(x)= \frac{p(x)}{q(x)} \newlineIf p(3)=2 p(3)= 2 , p′(3)=4 p'(3)= 4 , q(3)=6 q(3)= 6 , and q′(3)=9 q'(3)= 9 , what is r′(3) r'(3) ? \newlineSimplify any fractions. \newliner′(3)= r'(3)= _____
Get tutor helpright-arrow

Posted 7 months ago

Question
The functions u(x) u(x) and v(x) v(x) are differentiable. \newlineThe function w(x) w(x) is defined as: w(x)=u(x)v(x) w(x)= \frac{u(x)}{v(x)} \newlineIf u(5)=3 u(5)= 3 , u′(5)=−2 u'(5)= -2 , v(5)=7 v(5)= 7 , and v′(5)=1 v'(5)= 1 , what is w′(5) w'(5) ? \newlineSimplify any fractions. \newlinew′(5)= w'(5)= _____
Get tutor helpright-arrow

Posted 7 months ago

Question
The functions a(x) a(x) and b(x) b(x) are differentiable. \newlineThe function c(x) c(x) is defined as: c(x)=a(x)b(x) c(x)= \frac{a(x)}{b(x)} \newlineIf a(2)=4 a(2)= 4 , a′(2)=5 a'(2)= 5 , b(2)=1 b(2)= 1 , and b′(2)=−3 b'(2)= -3 , what is c′(2) c'(2) ? \newlineSimplify any fractions. \newlinec′(2)= c'(2)= _____
Get tutor helpright-arrow

Posted 7 months ago

Question
The functions m(x) m(x) and n(x) n(x) are differentiable. \newlineThe function o(x) o(x) is defined as: o(x)=m(x)n(x) o(x)= \frac{m(x)}{n(x)} \newlineIf m(7)=2 m(7)= 2 , m′(7)=−1 m'(7)= -1 , n(7)=4 n(7)= 4 , and n′(7)=8 n'(7)= 8 , what is o′(7) o'(7) ? \newlineSimplify any fractions. \newlineo′(7)= o'(7)= _____
Get tutor helpright-arrow

Posted 7 months ago

View all (427)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant