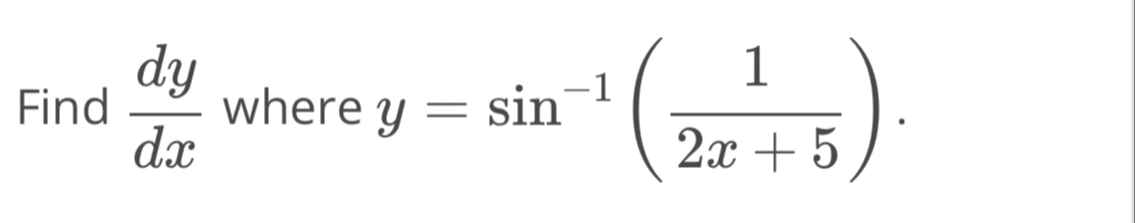Find (dy)/(dx) where y=sin^(-1)((1)/(2x+5)).

Question

Find  (dy)/(dx) where  y=sin^(-1)((1)/(2x+5)).

Bytelearn · Accepted Answer

Identify Functions: We need to find the derivative of y with respect to x, where y = sin^(-1)((1)/(2x+5)). To do this, we will use the chain rule, which states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function.Derivative of Outer Function: First, let's identify the outer function and the inner function. The outer function is sin^(-1)(u), where u is the inner function. The inner function is (1)/(2x+5).Derivative of Inner Function: The derivative of sin^(-1)(u) with respect to u is (1)/(sqrt(1-u^2)). So, we will need to substitute u with (1)/(2x+5) and then multiply by the derivative of the inner function.Combine Derivatives: The derivative of the inner function (1)/(2x+5) with respect to x is the derivative of a constant multiple of x plus a constant. The derivative of 1/(2x+5) is -1/(2x+5)^2 times the derivative of (2x+5), which is 2.Simplify Expression: Now we can combine the derivatives of the outer and inner functions. The derivative of y with respect to x is (1)/(sqrt(1-((1)/(2x+5))^2)) * (-2)/(2x+5)^2.Further Simplification: Simplify the expression by combining the constants and the terms with (2x+5). The derivative of y with respect to x is (-2)/(sqrt(1-((1)/(2x+5))^2) * (2x+5)^2).Common Denominator: We can further simplify the expression by squaring (1)/(2x+5) in the square root. This gives us sqrt(1-(1/(2x+5)^2)) in the denominator. The derivative of y with respect to x is (-2)/(sqrt(1-(1/(2x+5)^2)) * (2x+5)^2).Final Derivative: Finally, we can simplify the square root expression by finding a common denominator inside the square root. This gives us sqrt(((2x+5)^2-1)/(2x+5)^2). The derivative of y with respect to x is (-2)/(sqrt(((2x+5)^2-1)/(2x+5)^2) * (2x+5)^2).We can now simplify the expression by canceling out the (2x+5)^2 terms in the numerator and the denominator. The final derivative of y with respect to x is (-2)/sqrt((2x+5)^2-1).

Find $\frac{d y}{d x}$ where $y=\sin ^{-1}\left(\frac{1}{2 x+5}\right)$ .

Full solution

More problems from Evaluate rational exponents

Related topics

Find dydx \frac{d y}{d x} dxdy​ where y=sin⁡−1(12x+5) y=\sin ^{-1}\left(\frac{1}{2 x+5}\right) y=sin−1(2x+51​).

Find $\frac{d y}{d x}$ where $y=\sin ^{-1}\left(\frac{1}{2 x+5}\right)$ .