Full solution
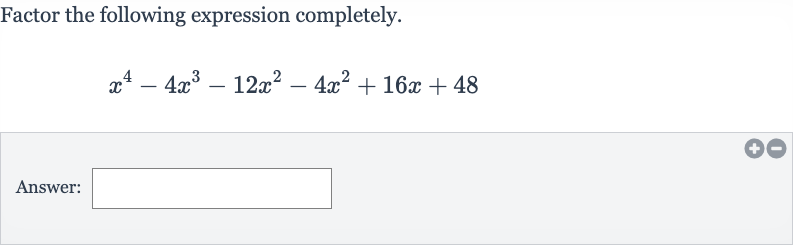
Q. Factor the following expression completely.Answer:
- Combine Like Terms: First, let's combine like terms in the expression.= =
- Find Common Factors: Now, we look for common factors in groups of terms.We can group the terms as follows: and .Let's factor out the greatest common factor from each group.For the first group, the greatest common factor is .For the second group, the greatest common factor is .So we have:
- Factor Quadratic Expression: Next, we factor the quadratic expression in the second group.The quadratic can be factored into .So the expression becomes:
- Check for Common Factor: Now, we look for a common factor in both groups.We notice that there is no common factor between and .Therefore, the expression is already factored completely.
More problems from Operations with rational exponents
QuestionGet tutor help
QuestionGet tutor help