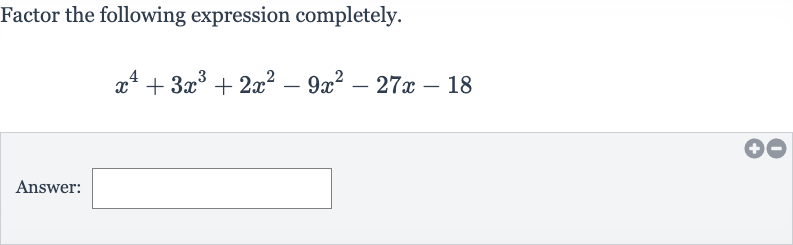
Factor the following expression completely. x^(4)+3x^(3)+2x^(2)-9x^(2)-27 x-18 Answer:

Question

Factor the following expression completely.  x^(4)+3x^(3)+2x^(2)-9x^(2)-27 x-18 Answer:

Bytelearn · Accepted Answer

Identify and group terms: Identify and group terms that can be combined in the polynomial. x^4 + 3x^3 + 2x^2 - 9x^2 - 27x - 18 can be rewritten by combining like terms (2x^2 - 9x^2). This gives us x^4 + 3x^3 - 7x^2 - 27x - 18.Look for common factors: Look for common factors in all terms. There are no common factors in all terms of the polynomial x^4 + 3x^3 - 7x^2 - 27x - 18.Group terms for factoring: Group terms to facilitate factoring by grouping. Group the terms as follows: (x^4 + 3x^3) + (-7x^2 - 27x) - 18.Factor by grouping: Factor by grouping. First group: x^4 + 3x^3 can be factored as x^3(x + 3). Second group: -7x^2 - 27x can be factored as -x(7x + 27). The expression becomes x^3(x + 3) - x(7x + 27) - 18.Notice common factor: Notice that the first and second groups have a common factor of x + 3. Rewrite the expression to make this common factor more apparent. The expression becomes x^3(x + 3) - 9x(x + 3) - 18.Factor out common factor: Factor out the common factor of x + 3. The expression becomes (x + 3)(x^3 - 9x) - 18.Factor further: Notice that x^3 - 9x can be factored further. x^3 - 9x is a difference of cubes since 9x = 3^2 * x and can be written as x(x^2 - 9). Factor x^2 - 9 as a difference of squares: x(x - 3)(x + 3). The expression becomes (x + 3)(x(x - 3)(x + 3)) - 18.Distribute x inside parentheses: Distribute the x inside the parentheses. The expression becomes (x + 3)(x^2(x - 3) + 3(x - 3)) - 18.Factor out common factor: Factor out the common factor of (x - 3) from the terms inside the parentheses. The expression becomes (x + 3)((x^2 + 3)(x - 3)) - 18.Distribute across: Distribute the (x + 3) across the (x^2 + 3)(x - 3). The expression becomes (x + 3)(x^2 + 3)(x - 3) - 18.Check for additional factor: Now, we need to see if -18 can be factored with the rest of the expression. Since there is no x term with -18, it cannot be factored with the rest of the expression. The final factored form of the expression is (x + 3)(x^2 + 3)(x - 3) - 18.

Factor the following expression completely. $\newline$ $x^{4}+3 x^{3}+2 x^{2}-9 x^{2}-27 x-18$ $\newline$ Answer:

Full solution

More problems from Operations with rational exponents

Related topics

Factor the following expression completely.\newlinex4+3x3+2x2−9x2−27x−18 x^{4}+3 x^{3}+2 x^{2}-9 x^{2}-27 x-18 x4+3x3+2x2−9x2−27x−18\newlineAnswer:

Factor the following expression completely. $\newline$ $x^{4}+3 x^{3}+2 x^{2}-9 x^{2}-27 x-18$ $\newline$ Answer: