Full solution
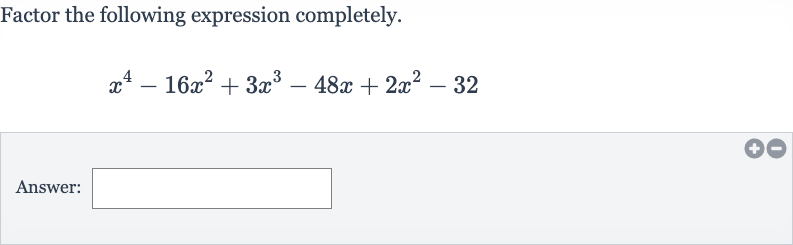
Q. Factor the following expression completely.Answer:
- Rearrange terms in descending order: First, we should rearrange the terms in descending order of the powers of to make it easier to factor.Combine like terms.
- Combine like terms: Next, we look for common factors in groups of terms. We can group the first three terms and the last three terms separately. -
- Group terms and find common factors: Now, factor by grouping.
- Factor by grouping: We can factor the quadratic .
- Factor quadratic expression: Now, we look for any common factors that can be factored out further, but there are none. So, the expression is completely factored.
More problems from Operations with rational exponents
QuestionGet tutor help
QuestionGet tutor help