AI tutor
Full solution
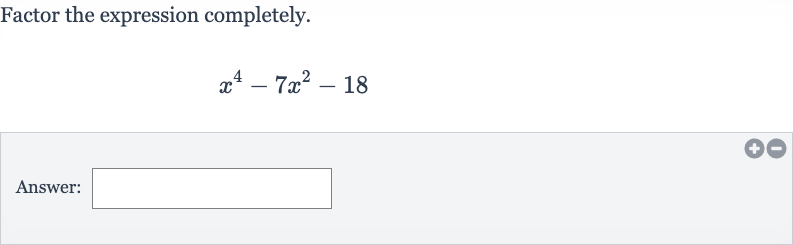
Q. Factor the expression completely.Answer:
- Identify type of polynomial: Identify the type of polynomial and look for a strategy to factor it. The given expression is a quadratic in form, with as the variable instead of . We can use substitution to make it look like a standard quadratic equation. Let , then the expression becomes .
- Factor quadratic expression: Factor the quadratic expression . We look for two numbers that multiply to and add up to . These numbers are and .
- Write factored form: Write the factored form of the quadratic using the numbers found in the previous step: .
- Substitute back in for u: Substitute back in for to get the factored form in terms of : .
- Factor difference of squares: Notice that is a difference of squares and can be factored further into .
- Combine fully factored terms: Combine the fully factored terms to get the final factored expression: .
More problems from Operations with rational exponents
QuestionGet tutor help
QuestionGet tutor help