Full solution
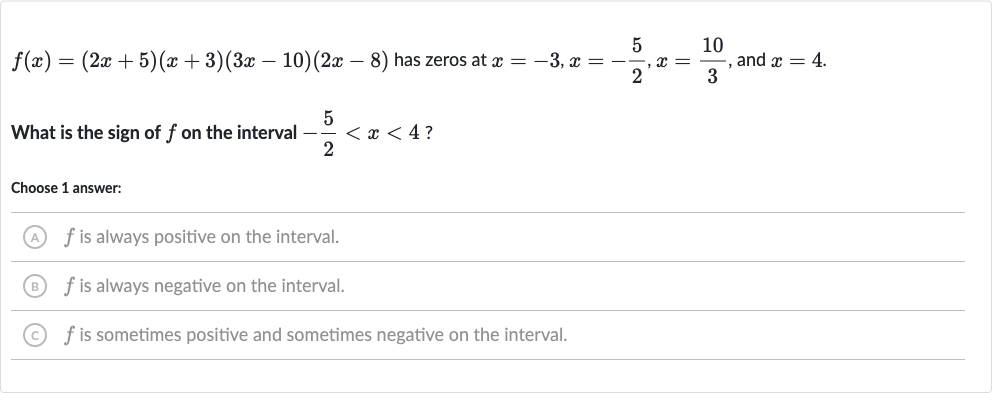
Q. has zeros at , and .What is the sign of on the interval ?Choose answer:(A) is always positive on the interval.(B) is always negative on the interval.(C) is sometimes positive and sometimes negative on the interval.
- Factor Analysis: To determine the sign of on the interval, we need to look at the sign of each factor in the given interval.
- Sign Determination: For the factor , when is between and , this factor is positive because is greater than zero for all in this interval.
- Factor : For the factor , when is between and , this factor is also positive because is greater than zero for all in this interval.
- Factor : For the factor , when is between and , this factor is negative because is less than zero when is less than , which is part of our interval.
- Factor : For the factor , when is between and , this factor is negative because is less than zero when is less than , which is part of our interval.
- Factor : Since we have two negative factors and two positive factors, the negatives will cancel each other out, and the overall sign of on the interval will be positive.