Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

A complex number
z_(1) has a magnitude
|z_(1)|=3 and an angle
theta_(1)=20^(@).
Express
z_(1) in rectangular form, as
z_(1)=a+bi.
Round
a and
b to the nearest thousandth.

z_(1)=◻+◻i

A complex number $z_{1}$ has a magnitude $\left|z_{1}\right|=3$ and an angle $\theta_{1}=20^{\circ}$ . $\newline$ Express $z_{1}$ in rectangular form, as $z_{1}=a+b i$ . $\newline$ Round $a$ and $b$ to the nearest thousandth. $\newline$ $z_{1}=\square+\square i$

View step-by-step help

View step-by-step help

Compare linear and exponential growth

Full solution

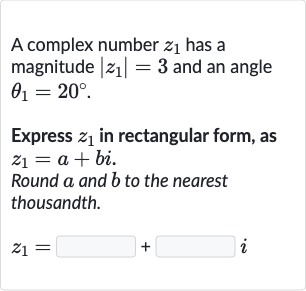
Q. A complex number

z_{1}

has a magnitude

\left|z_{1}\right|=3

and an angle

\theta_{1}=20^{\circ}

.

\newline

Express

z_{1}

in rectangular form, as

z_{1}=a+b i

.

\newline

Round

a

and

b

to the nearest thousandth.

\newline

z_{1}=\square+\square i

Convert to rectangular form: Convert the polar form of the complex number to rectangular form using the formula $z = r(\cos(\theta) + i\sin(\theta))$ , where $r$ is the magnitude and $\theta$ is the angle. $\newline$ Given: $|z_{1}| = 3$ and $\theta_{1} = 20$ degrees. $\newline$ We need to calculate the cosine and sine of $20$ degrees.
Calculate cosine and sine: Calculate the cosine and sine of $20$ degrees. $\newline$ Using a calculator or trigonometric tables: $\newline$ $\cos(20^\circ) \approx 0.9397$ $\newline$ $\sin(20^\circ) \approx 0.3420$
Multiply by magnitude: Multiply the cosine and sine values by the magnitude to get the real and imaginary parts of the complex number. $\newline$ $a = r \cdot \cos(\theta) = 3 \cdot 0.9397 \approx 2.8191$ $\newline$ $b = r \cdot \sin(\theta) = 3 \cdot 0.3420 \approx 1.0260$
Round to nearest thousandth: Round the real and imaginary parts to the nearest thousandth. $\newline$ $a \approx 2.819$ $\newline$ $b \approx 1.026$
Express in rectangular form: Express $z_{1}$ in rectangular form using the rounded values of $a$ and $b$ . $z_{1} = a + bi = 2.819 + 1.026i$

More problems from Compare linear and exponential growth

Question

Jill bought a used motorcycle from a seller online for $1,200. The seller will charge her $5 a day to store the motorcycle at his house until she is able to pick it up. You can use a function to describe the total amount of money Jill will owe the seller if she waits

x

days to pick up the motorcycle.

\newline

Write an equation for the function. If it is linear, write it in the form

g(x) = mx + b

. If it is exponential, write it in the form

g(x) = a(b)^x

\newline

g(x) = \underline{\hspace{3em}}

Posted 5 months ago

Question

f(t)=-2t-7

change over the interval from

t=-7

t=-6

\newline

\newline

\left[\text{f(t) decreases by } 2\right]

\newline

\left[\text{f(t) increases by } 2\right]

\newline

\left[\text{f(t) increases by } 200\%\right]

\newline

\left[\text{f(t) decreases by a factor of } 2\right]

Posted 5 months ago

Question

g(t)=9^t

change over the interval from

t=1

t=3

\newline

\newline

\left[\text{g(t) decreases by a factor of } 81\right]

\newline

\left[\text{g(t) increases by a factor of } 81\right]

\newline

\left[\text{g(t) increases by } 18\%\right]

\newline

\left[\text{g(t) decreases by } 9\%\right]

Posted 5 months ago

Question

Both of these functions grow as

x

gets larger and larger. Which function eventually exceeds the other?

\newline

\newline

(A)f(x) = 8x + 3.3

\newline

(B)g(x) = 3.3^x - 5

Posted 6 months ago

Question

Both of these functions grow as

x

gets larger and larger. Which function eventually exceeds the other?

\newline

\newline

(A) \ f(x) = 2x + 9

\newline

(B)\ g(x) = 2^x

Posted 6 months ago

Question

f(x)

g(x)

are differentiable.

\newline

h(x)

h(x)=\frac{g(x)}{f(x)}

\newline

f(8)=1

f'(8)=-6

g(8)=8

g'(8)=-10

h'(8)

\newline

Simplify any fractions.

\newline

h'(8)=

Posted 6 months ago

Question

p(x)

q(x)

are differentiable.

\newline

r(x)

r(x)= \frac{p(x)}{q(x)}

\newline

p(3)= 2

p'(3)= 4

q(3)= 6

q'(3)= 9

r'(3)

\newline

Simplify any fractions.

\newline

r'(3)=

Posted 7 months ago

Question

u(x)

v(x)

are differentiable.

\newline

w(x)

w(x)= \frac{u(x)}{v(x)}

\newline

u(5)= 3

u'(5)= -2

v(5)= 7

v'(5)= 1

w'(5)

\newline

Simplify any fractions.

\newline

w'(5)=

Posted 7 months ago

Question

a(x)

b(x)

are differentiable.

\newline

c(x)

c(x)= \frac{a(x)}{b(x)}

\newline

a(2)= 4

a'(2)= 5

b(2)= 1

b'(2)= -3

c'(2)

\newline

Simplify any fractions.

\newline

c'(2)=

Posted 7 months ago

Question

m(x)

n(x)

are differentiable.

\newline

o(x)

o(x)= \frac{m(x)}{n(x)}

\newline

m(7)= 2

m'(7)= -1

n(7)= 4

n'(7)= 8

o'(7)

\newline

Simplify any fractions.

\newline

o'(7)=

Posted 7 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant