Full solution
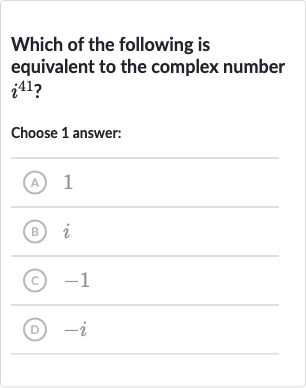
Q. Which of the following is equivalent to the complex number ?Choose answer:(A) (B) (C) (D)
- Definition of i: To find the equivalent of i raised to any power, we need to remember that i is the imaginary unit, defined as . The powers of i repeat in a cycle: , , , , and then back to . This cycle repeats every powers.
- Determining the Power Cycle: Let's find the remainder when is divided by to determine where in the cycle will land. divided by is with a remainder of .
- Finding the Remainder: Since the remainder is , is equivalent to , which is the same as . We know that , so this simplifies to , which is just .
- Simplifying the Expression: Therefore, is equivalent to . This corresponds to choice in the given options.
More problems from Domain and range of quadratic functions: equations
QuestionGet tutor help