Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

10 rubber stamps cost
$10.30.
Which equation would help determine the cost of 2 rubber stamps?
Choose 1 answer:
(A)
(2)/(x)=($10.30)/(10)
(B)
(x)/(2)=(10)/($10.30)
(c)
(2)/(10)=($10.30)/(x)
(D)
(2)/($10.30)=(x)/( 10)
(E) None of the above

$10$ rubber stamps cost $\$ 10.30$ . $\newline$ Which equation would help determine the cost of $2$ rubber stamps? $\newline$ Choose $1$ answer: $\newline$ (A) $\frac{2}{x}=\frac{\$ 10.30}{10}$ $\newline$ (B) $\frac{x}{2}=\frac{10}{\$ 10.30}$ $\newline$ (C) $\frac{2}{10}=\frac{\$ 10.30}{x}$ $\newline$ (D) $\frac{2}{\$ 10.30}=\frac{x}{10}$ $\newline$ (E) None of the above

View step-by-step help

View step-by-step help

Compare linear and exponential growth

Full solution

Q.

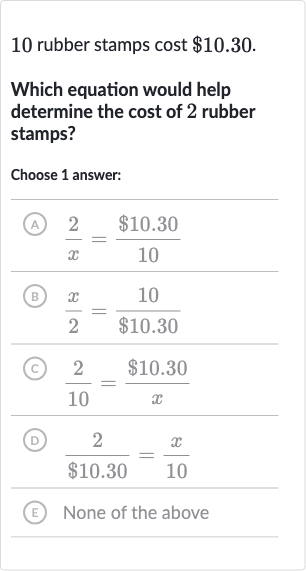
10

rubber stamps cost

\$ 10.30

.

\newline

Which equation would help determine the cost of

2

rubber stamps?

\newline

Choose

1

answer:

\newline

(A)

\frac{2}{x}=\frac{\$ 10.30}{10}

\newline

(B)

\frac{x}{2}=\frac{10}{\$ 10.30}

\newline

(C)

\frac{2}{10}=\frac{\$ 10.30}{x}

\newline

(D)

\frac{2}{\$ 10.30}=\frac{x}{10}

\newline

(E) None of the above

Denote Cost as $x$ : Let's denote the cost of one rubber stamp as $x$ dollars. We know that $10$ rubber stamps cost $\$10.30$ . We want to find an equation that relates the cost of $2$ rubber stamps to the cost of $10$ rubber stamps. The direct proportionality between the number of stamps and the total cost suggests that we can set up a ratio comparing the cost of $2$ stamps to the cost of $10$ stamps.
Set Up Proportion: We can write the proportion as $(\text{cost of } 2 \text{ stamps})/(\text{cost of } 10 \text{ stamps}) = (\text{number of stamps for } 2)/(\text{number of stamps for } 10)$ . This simplifies to $(2x)/(10.30) = 2/10$ . We want to solve for $x$ , the cost of $2$ stamps.
Cross-Multiply to Solve: To solve for $x$ , we can cross-multiply to get $2 \times 10.30 = 2x \times 10$ . This simplifies to $20.60 = 20x$ . Now we can divide both sides by $20$ to find the value of $x$ .
Calculate Cost of $2$ Stamps: Dividing both sides of the equation $20.60 = 20x$ by $20$ gives us $x = \frac{20.60}{20}$ . This simplifies to $x = 1.03$ . Therefore, the cost of $2$ rubber stamps is $2 \times 1.03 = \$(2.06)$ .
Match Proportion to Choices: Now we need to match our proportion to the given answer choices. The correct proportion that we used is $(2x)/(10.30) = 2/10$ , which simplifies to $x = 1.03$ , the cost of $2$ stamps. This proportion is represented by choice (C) $(2)/(10)=(\$(10.30))/(x)$ .

More problems from Compare linear and exponential growth

Question

Jill bought a used motorcycle from a seller online for $1,200. The seller will charge her $5 a day to store the motorcycle at his house until she is able to pick it up. You can use a function to describe the total amount of money Jill will owe the seller if she waits

x

days to pick up the motorcycle.

\newline

Write an equation for the function. If it is linear, write it in the form

g(x) = mx + b

. If it is exponential, write it in the form

g(x) = a(b)^x

\newline

g(x) = \underline{\hspace{3em}}

Posted 5 months ago

Question

f(t)=-2t-7

change over the interval from

t=-7

t=-6

\newline

\newline

\left[\text{f(t) decreases by } 2\right]

\newline

\left[\text{f(t) increases by } 2\right]

\newline

\left[\text{f(t) increases by } 200\%\right]

\newline

\left[\text{f(t) decreases by a factor of } 2\right]

Posted 5 months ago

Question

g(t)=9^t

change over the interval from

t=1

t=3

\newline

\newline

\left[\text{g(t) decreases by a factor of } 81\right]

\newline

\left[\text{g(t) increases by a factor of } 81\right]

\newline

\left[\text{g(t) increases by } 18\%\right]

\newline

\left[\text{g(t) decreases by } 9\%\right]

Posted 5 months ago

Question

Both of these functions grow as

x

gets larger and larger. Which function eventually exceeds the other?

\newline

\newline

(A)f(x) = 8x + 3.3

\newline

(B)g(x) = 3.3^x - 5

Posted 6 months ago

Question

Both of these functions grow as

x

gets larger and larger. Which function eventually exceeds the other?

\newline

\newline

(A) \ f(x) = 2x + 9

\newline

(B)\ g(x) = 2^x

Posted 6 months ago

Question

f(x)

g(x)

are differentiable.

\newline

h(x)

h(x)=\frac{g(x)}{f(x)}

\newline

f(8)=1

f'(8)=-6

g(8)=8

g'(8)=-10

h'(8)

\newline

Simplify any fractions.

\newline

h'(8)=

Posted 6 months ago

Question

p(x)

q(x)

are differentiable.

\newline

r(x)

r(x)= \frac{p(x)}{q(x)}

\newline

p(3)= 2

p'(3)= 4

q(3)= 6

q'(3)= 9

r'(3)

\newline

Simplify any fractions.

\newline

r'(3)=

Posted 7 months ago

Question

u(x)

v(x)

are differentiable.

\newline

w(x)

w(x)= \frac{u(x)}{v(x)}

\newline

u(5)= 3

u'(5)= -2

v(5)= 7

v'(5)= 1

w'(5)

\newline

Simplify any fractions.

\newline

w'(5)=

Posted 7 months ago

Question

a(x)

b(x)

are differentiable.

\newline

c(x)

c(x)= \frac{a(x)}{b(x)}

\newline

a(2)= 4

a'(2)= 5

b(2)= 1

b'(2)= -3

c'(2)

\newline

Simplify any fractions.

\newline

c'(2)=

Posted 7 months ago

Question

m(x)

n(x)

are differentiable.

\newline

o(x)

o(x)= \frac{m(x)}{n(x)}

\newline

m(7)= 2

m'(7)= -1

n(7)= 4

n'(7)= 8

o'(7)

\newline

Simplify any fractions.

\newline

o'(7)=

Posted 7 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant