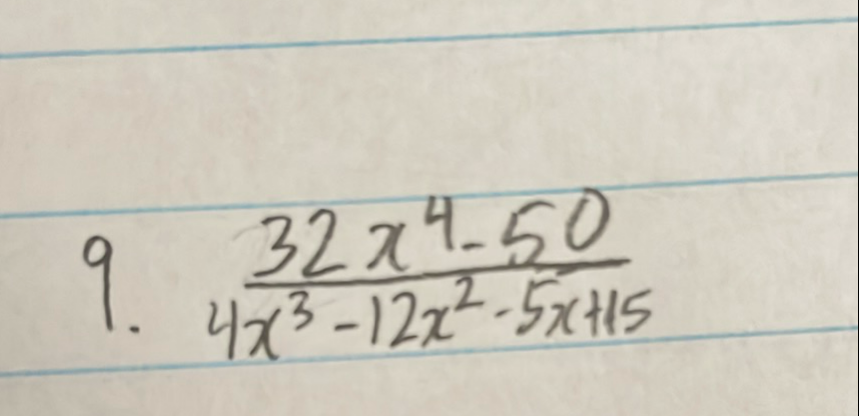(32x^(4)-50)/(4x^(3)-12x^(2)-5x+15)

Question

Bytelearn · Accepted Answer

Identify Common Factors: Identify if the numerator and denominator have any common factors that can be factored out. The numerator is 32x^4 - 50, which can be factored as 2(16x^4 - 25). The denominator is 4x^3 - 12x^2 - 5x + 15, which does not have an obvious common factor.Factorize Numerator: Check if the numerator can be factored further. The expression 16x^4 - 25 is a difference of squares and can be factored as (4x^2 + 5)(4x^2 - 5).Factorize Denominator: Check if the denominator can be factored by grouping. Group the terms as (4x^3 - 12x^2) + (-5x + 15) and factor out the common factors. This gives us 4x^2(x - 3) - 5(x - 3). Now we can factor out (x - 3) to get (x - 3)(4x^2 - 5).Simplify Expression: Now that we have factored both the numerator and the denominator, we can simplify the expression. The simplified form is (2(4x^2 + 5)(4x^2 - 5)) / ((x - 3)(4x^2 - 5)). We can cancel out the common factor of (4x^2 - 5).Final Simplified Form: After canceling the common factor, we are left with the simplified expression. The final simplified form is 2(4x^2 + 5) / (x - 3).

$\frac{32 x^{4}-50}{4 x^{3}-12 x^{2}-5 x+15}$

Full solution

More problems from Operations with rational exponents

Related topics

32x4−504x3−12x2−5x+15 \frac{32 x^{4}-50}{4 x^{3}-12 x^{2}-5 x+15} 4x3−12x2−5x+1532x4−50​

$\frac{32 x^{4}-50}{4 x^{3}-12 x^{2}-5 x+15}$