Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

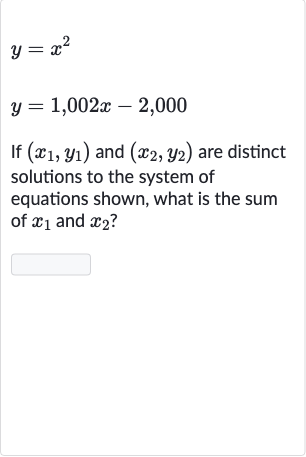
y=x^(2)

y=1,002 x-2,000
If
(x_(1),y_(1)) and
(x_(2),y_(2)) are distinct solutions to the system of equations shown, what is the sum of
x_(1) and
x_(2) ?

$y=x^{2}$ $\newline$ $y=1,002x-2,000$ $\newline$ If $\newline$ $(x_{1},y_{1})$ and $\newline$ $(x_{2},y_{2})$ are distinct solutions to the system of equations shown, what is the sum of $\newline$ $x_{1}$ and $\newline$ $x_{2}$ ?

View step-by-step help

View step-by-step help

Evaluate expression when two complex numbers are given

Full solution

Q.

y=x^{2}

\newline

y=1,002x-2,000

\newline

If

\newline

(x_{1},y_{1})

and

\newline

(x_{2},y_{2})

are distinct solutions to the system of equations shown, what is the sum of

\newline

x_{1}

and

\newline

x_{2}

?

Set Equations Equal: We are given the system of equations: $\newline$ $y = x^2$ $\newline$ $y = 1,002x - 2,000$ $\newline$ To find the $x$ -coordinates of the solutions, we need to set the two equations equal to each other because they both equal $y$ . $\newline$ $x^2 = 1,002x - 2,000$
Rearrange to Quadratic Form: Now we need to solve for $x$ . To do this, we will rearrange the equation into a standard quadratic form. $x^2 - 1,002x + 2,000 = 0$
Apply Quadratic Formula: To find the solutions for $x$ , we can use the quadratic formula: $x = \frac{-b \pm \sqrt{b^2 - 4ac}}{2a}$ , where $a = 1$ , $b = -1,002$ , and $c = 2,000$ . However, we are asked for the sum of the solutions, which according to Vieta's formulas, is equal to $-\frac{b}{a}$ .
Use Vieta's Formulas: Using Vieta's formulas, the sum of the solutions for the quadratic equation $ax^2 + bx + c = 0$ is $-\frac{b}{a}$ . So, the sum of $x_{1}$ and $x_{2}$ is $-\frac{-1,002}{1}.$
Calculate the Sum: Calculating the sum gives us: $\newline$ Sum = $\frac{1,002}{1}$ $\newline$ Sum = $1,002$

More problems from Evaluate expression when two complex numbers are given

Question

What is the period of

y=3 \cos (x-7)+2

\newline

Give an exact value.

\newline

Posted 10 months ago

Question

(-10 i)+(-40+8 i)=

\newline

Express your answer in the form

(a+b i)

Posted 10 months ago

Question

(8-i)-(-82+2 i)=

\newline

Express your answer in the form

(a+b i)

Posted 10 months ago

Question

(80 i)-(80+10 i)=

\newline

Express your answer in the form

(a+b i)

Posted 10 months ago

Question

Express the radical using the imaginary unit,

i

\newline

Express your answer in simplified form.

\newline

\pm \sqrt{-64}= \pm

Posted 10 months ago

Question

Which of the following is equivalent to the complex number

i^{21}

\newline

1

\newline

1

\newline

i

\newline

-1

\newline

-i

Posted 10 months ago

Question

Express the radical using the imaginary unit,

i

\newline

Express your answer in simplified form.

\newline

\pm \sqrt{-30}= \pm

Posted 10 months ago

Question

Express the radical using the imaginary unit,

i

\newline

Express your answer in simplified form.

\newline

\pm \sqrt{-35}= \pm

Posted 10 months ago

Question

Express the radical using the imaginary unit,

i

\newline

Express your answer in simplified form.

\newline

\pm \sqrt{-80}= \pm

Posted 10 months ago

Question

Express the radical using the imaginary unit,

i

\newline

Express your answer in simplified form.

\newline

\pm \sqrt{-4}= \pm

Posted 10 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant