Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

y=arctan(-4x)
Evaluate
(dy)/(dx) at
x=3.
Use an exact expression.

$y=\arctan (-4 x)$ $\newline$ Evaluate $\frac{d y}{d x}$ at $x=3$ . $\newline$ Use an exact expression.

View step-by-step help

View step-by-step help

Find derivatives of inverse trigonometric functions

Full solution

Q.

y=\arctan (-4 x)

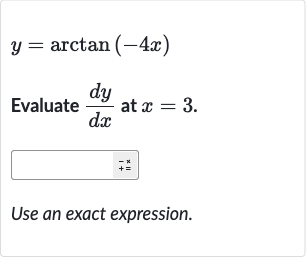
\newline

Evaluate

\frac{d y}{d x}

at

x=3

.

\newline

Use an exact expression.

Differentiate using chain rule: step_1: Differentiate $y = \arctan(-4x)$ with respect to $x$ using the chain rule. $\newline$ The derivative of $\arctan(u)$ with respect to $u$ is $\frac{1}{1+u^2}$ , so by the chain rule, $\frac{dy}{dx} = \left(\frac{1}{1+(-4x)^2}\right) * \frac{d(-4x)}{dx}$ .
Calculate derivative of $-4x$ : step_2: Calculate the derivative of $-4x$ with respect to $x$ . $\frac{d(-4x)}{dx} = -4.$
Substitute derivative into result: step_3: Substitute the derivative of $-4x$ into the result from step $1$ . $\newline$ \frac{dy}{dx} = \left(\frac{1}{1+(\-4x)^2}\right) * (\-4).
Simplify expression for $\frac{dy}{dx}$ : step_4: Simplify the expression for $\frac{dy}{dx}$ . $\frac{dy}{dx} = \frac{-4}{1+16x^2}$ .
Evaluate $\frac{dy}{dx}$ at $x = 3$ : step_5: Evaluate $\frac{dy}{dx}$ at $x = 3$ . $\frac{dy}{dx}$ at $x = 3$ is $-\frac{4}{1+16(3)^2}$ .
Calculate exact expression at $x = 3$ : step_6: Calculate the exact expression for $\frac{dy}{dx}$ at $x = 3$ . $\frac{dy}{dx}$ at $x = 3$ is $-\frac{4}{1+16\cdot 9}$ .
Simplify the denominator: step_7: Simplify the denominator. $\newline$ $1+16\times 9 = 1+144$ .
Add numbers in the denominator: step_8: Add the numbers in the denominator. $1+144 = 145$ .
Write final expression for $\frac{dy}{dx}$ : step_9: Write the final expression for $\frac{dy}{dx}$ at $x = 3$ . $\newline$ $\frac{dy}{dx}$ at $x = 3$ is $-\frac{4}{145}$ .

More problems from Find derivatives of inverse trigonometric functions

Question

\lim_{\theta \rightarrow \frac{\pi}{2}} \tan ^{2}(\theta)[1-\sin (\theta)]

\newline

1

\newline

0

\newline

\frac{1}{2}

\newline

-2

\newline

(D) The limit doesn't exist

Posted 3 months ago

Question

\lim _{\theta \rightarrow \frac{\pi}{2}} \frac{\sin ^{2}(2 \theta)}{1-\sin ^{2}(\theta)}

\newline

1

\newline

1

\newline

2

\newline

4

\newline

(D) The limit doesn't exist

Posted 3 months ago

Question

\lim _{x \rightarrow 3} \frac{x-3}{\sqrt{4 x+4}-4}

\newline

1

\newline

-4

\newline

1

\newline

2

\newline

(D) The limit doesn't exist

Posted 3 months ago

Question

\lim _{x \rightarrow-4} \frac{7 x+28}{x^{2}+x-12}

\newline

1

\newline

1

\newline

7

\newline

-1

\newline

(D) The limit doesn't exist

Posted 3 months ago

Question

\lim _{x \rightarrow-3} \frac{x+3}{4-\sqrt{2 x+22}}

\newline

1

\newline

-3

\newline

-4

\newline

-\frac{3}{4}

\newline

(D) The limit doesn't exist

Posted 3 months ago

Question

\lim _{x \rightarrow 1} \frac{\sqrt{5 x+4}-3}{x-1}

\newline

1

\newline

\frac{3}{5}

\newline

\frac{5}{6}

\newline

1

\newline

(D) The limit doesn't exist

Posted 3 months ago

Question

\lim _{x \rightarrow-2} \frac{x^{3}+3 x^{2}+2 x}{x+2}

\newline

1

\newline

6

\newline

0

\newline

2

\newline

(D) The limit doesn't exist

Posted 3 months ago

Question

\lim _{x \rightarrow \frac{\pi}{2}} \frac{\cot ^{2}(x)}{1-\sin (x)}

\newline

1

\newline

-1

\newline

-\frac{\pi}{2}

\newline

2

\newline

(D) The limit doesn't exist

Posted 3 months ago

Question

\lim _{x \rightarrow \frac{\pi}{2}} \frac{\sin (2 x)}{\cos (x)}

\newline

1

\newline

\frac{1}{2}

\newline

1

\newline

2

\newline

(D) The limit doesn't exist

Posted 3 months ago

Question

\lim _{\theta \rightarrow \frac{\pi}{4}} \frac{\cos (2 \theta)}{\sqrt{2} \cos (\theta)-1}

\newline

1

\newline

2

\newline

\frac{1}{2}

\newline

\sqrt{2}

\newline

(D) The limit doesn't exist

Posted 3 months ago