$y=\arccos \left(-\frac{x}{5}\right)$ $\newline$ Evaluate $\frac{d y}{d x}$ at $x=2$ . $\newline$ Use an exact expression.

View step-by-step help

Home

Math Problems

Calculus

Find derivatives of inverse trigonometric functions

Full solution

y=\arccos \left(-\frac{x}{5}\right)

\newline

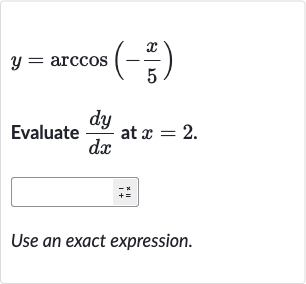
Evaluate

\frac{d y}{d x}

x=2

\newline

Use an exact expression.

Find Derivative of $y=\arccos\left(-\frac{x}{5}\right):</b> First, let's find the derivative of \$y=\arccos\left(-\frac{x}{5}\right)$ . Using the chain rule, the derivative of $\arccos(u)$ is $-\frac{1}{\sqrt{1-u^2}}$ times the derivative of $u$ .
Apply Chain Rule: Let $u=-\frac{x}{5}$ . Then, $\frac{du}{dx} = -\frac{1}{5}$ .
Plug in $u$ and Simplify: Now, plug $u$ into the derivative of $\arccos(u)$ to get $\frac{dy}{dx} = -\frac{1}{\sqrt{1-\left(-\frac{x}{5}\right)^2}} \cdot \left(-\frac{1}{5}\right)$ .
Evaluate at $x=2$ : Simplify the expression: $\frac{dy}{dx} = -\frac{1}{\sqrt{1-\left(\frac{x^2}{25}\right)}} \cdot \left(-\frac{1}{5}\right)$ .
Calculate Inside Square Root: Now, evaluate $\frac{dy}{dx}$ at $x=2$ . Plug $x=2$ into the derivative: $\frac{dy}{dx} = -\frac{1}{\sqrt{1-\left(\frac{2^2}{25}\right)}} \times \left(-\frac{1}{5}\right)$ .
Take Square Root: Calculate the inside of the square root: $1-(2^2)/(25) = 1-(4)/(25) = 1-4/25 = 21/25$ .
Multiply by $-\frac{1}{5}$ : Now, take the square root: $\sqrt{\frac{21}{25}} = \frac{\sqrt{21}}{5}$ .
Multiply by $-\frac{1}{5}$ : Now, take the square root: $\sqrt{\frac{21}{25}} = \frac{\sqrt{21}}{5}$ .Finally, multiply by $-\frac{1}{5}$ : $\frac{dy}{dx} = -\frac{1}{\sqrt{21}/5} \times (-\frac{1}{5}) = \frac{1}{5\sqrt{21}/5} = \frac{1}{\sqrt{21}}$ .

More problems from Find derivatives of inverse trigonometric functions

Question
Find $\lim_{\theta \rightarrow \frac{\pi}{2}} \tan ^{2}(\theta)[1-\sin (\theta)]$ . $\newline$ Choose $1$ answer: $\newline$ (A) $0$ $\newline$ (B) $\frac{1}{2}$ $\newline$ (C) $-2$ $\newline$ (D) The limit doesn't exist
Get tutor help
Posted 3 months ago

Question
Find $\lim _{\theta \rightarrow \frac{\pi}{2}} \frac{\sin ^{2}(2 \theta)}{1-\sin ^{2}(\theta)}$ $\newline$ Choose $1$ answer: $\newline$ (A) $1$ $\newline$ (B) $2$ $\newline$ (C) $4$ $\newline$ (D) The limit doesn't exist
Get tutor help
Posted 3 months ago

Question
Find $\lim _{x \rightarrow 3} \frac{x-3}{\sqrt{4 x+4}-4}$ . $\newline$ Choose $1$ answer: $\newline$ (A) $-4$ $\newline$ (B) $1$ $\newline$ (C) $2$ $\newline$ (D) The limit doesn't exist
Get tutor help
Posted 3 months ago

Question
Find $\lim _{x \rightarrow-4} \frac{7 x+28}{x^{2}+x-12}$ . $\newline$ Choose $1$ answer: $\newline$ (A) $1$ $\newline$ (B) $7$ $\newline$ (C) $-1$ $\newline$ (D) The limit doesn't exist
Get tutor help
Posted 3 months ago

Question
Find $\lim _{x \rightarrow-3} \frac{x+3}{4-\sqrt{2 x+22}}$ . $\newline$ Choose $1$ answer: $\newline$ (A) $-3$ $\newline$ (B) $-4$ $\newline$ (C) $-\frac{3}{4}$ $\newline$ (D) The limit doesn't exist
Get tutor help
Posted 3 months ago

Question
Find $\lim _{x \rightarrow 1} \frac{\sqrt{5 x+4}-3}{x-1}$ . $\newline$ Choose $1$ answer: $\newline$ (A) $\frac{3}{5}$ $\newline$ (B) $\frac{5}{6}$ $\newline$ (C) $1$ $\newline$ (D) The limit doesn't exist
Get tutor help
Posted 3 months ago

Question
Find $\lim _{x \rightarrow-2} \frac{x^{3}+3 x^{2}+2 x}{x+2}$ . $\newline$ Choose $1$ answer: $\newline$ (A) $6$ $\newline$ (B) $0$ $\newline$ (C) $2$ $\newline$ (D) The limit doesn't exist
Get tutor help
Posted 3 months ago

Question
Find $\lim _{x \rightarrow \frac{\pi}{2}} \frac{\cot ^{2}(x)}{1-\sin (x)}$ $\newline$ Choose $1$ answer: $\newline$ (A) $-1$ $\newline$ (B) $-\frac{\pi}{2}$ $\newline$ (C) $2$ $\newline$ (D) The limit doesn't exist
Get tutor help
Posted 3 months ago

Question
Find $\lim _{x \rightarrow \frac{\pi}{2}} \frac{\sin (2 x)}{\cos (x)}$ . $\newline$ Choose $1$ answer: $\newline$ (A) $\frac{1}{2}$ $\newline$ (B) $1$ $\newline$ (C) $2$ $\newline$ (D) The limit doesn't exist
Get tutor help
Posted 3 months ago

Question
Find $\lim _{\theta \rightarrow \frac{\pi}{4}} \frac{\cos (2 \theta)}{\sqrt{2} \cos (\theta)-1}$ . $\newline$ Choose $1$ answer: $\newline$ (A) $2$ $\newline$ (B) $\frac{1}{2}$ $\newline$ (C) $\sqrt{2}$ $\newline$ (D) The limit doesn't exist
Get tutor help
Posted 3 months ago

View all (16)

y=arccos⁡(−x5) y=\arccos \left(-\frac{x}{5}\right) y=arccos(−5x​)\newlineEvaluate dydx \frac{d y}{d x} dxdy​ at x=2 x=2 x=2.\newlineUse an exact expression.

$y=\arccos \left(-\frac{x}{5}\right)$ $\newline$ Evaluate $\frac{d y}{d x}$ at $x=2$ . $\newline$ Use an exact expression.