Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

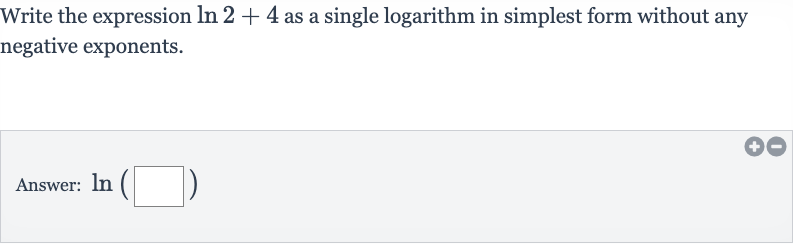
Write the expression
ln 2+4 as a single logarithm in simplest form without any negative exponents.
Answer:
ln(◻)

Write the expression $\ln 2+4$ as a single logarithm in simplest form without any negative exponents. $\newline$ Answer: $\ln (\square)$

View step-by-step help

View step-by-step help

Simplify radical expressions with variables

Full solution

Q. Write the expression

\ln 2+4

as a single logarithm in simplest form without any negative exponents.

\newline

Answer:

\ln (\square)

Understand Logarithm Properties: Understand the properties of logarithms The problem asks us to combine $\ln 2$ and $4$ into a single logarithm. To do this, we need to use the properties of logarithms. One of the properties is that the logarithm of a product is the sum of the logarithms ( $\log(a) + \log(b) = \log(ab)$ ). We will use this property to combine $\ln 2$ and $4$ into a single logarithm.
Convert Number $4$ to Logarithm: Convert the number $4$ into a logarithm with the same base To use the property from Step $1$ , we need to express the number $4$ as a logarithm with the same base, which is the natural logarithm (base $e$ ). We can write $4$ as $\ln(e^4)$ because the natural logarithm of $e$ to any power is just that power ( $\ln(e^x) = x$ ).
Combine Logarithms: Combine the two logarithms into one $\newline$ Now that we have both numbers as natural logarithms, we can combine them using the property from Step $1$ : $\newline$ $\ln 2 + \ln(e^4) = \ln(2 \cdot e^4)$
Simplify Expression: Simplify the expression The expression $\ln(2 \cdot e^4)$ is already in its simplest form. There are no negative exponents, and it is written as a single logarithm.

More problems from Simplify radical expressions with variables

Question

\newline

500

=

Posted 9 months ago

Question

\newline

2,000

=

Posted 9 months ago

Question

\newline

750

=

Posted 9 months ago

Question

\newline

1,500

=

Posted 9 months ago

Question

\newline

1,500

=

Posted 9 months ago

Question

According to Newton's Second Law of Motion, the sum of the forces that act on an object with a mass

m

that moves with an acceleration

a

m \cdot a

. An object whose mass is

80

grams has an acceleration of

20

meters per seconds squared. What calculation will give us the sum of the forces that act on the object, in Newtons (which are

\frac{\text{kg} \cdot \text{m}}{\text{s}^2}

\newline

1

\newline

80 \cdot 20

\newline

80 \cdot 1000 \cdot 20

\newline

\frac{80}{1000} \cdot 20

\newline

\frac{80}{60^2} \cdot 20

Posted 8 months ago

Question

\newline

Multiply and remove all perfect squares from inside the square roots. Assume

\newline

b

\newline

2\sqrt{8b^{3}} \cdot 9\sqrt{18b} =

Posted 10 months ago

Question

Roselyn is driving to visit her family, who live

150

kilometers away. Her average speed is

60

kilometers per hour. The car's tank has

20

liters of fuel at the beginning of the drive, and its fuel efficiency is

6

kilometers per liter. Fuel costs

0.60

dollars per liter.

\newline

How long can Roselyn drive before she runs out of fuel?

\newline

Posted 10 months ago

Question

Astrid is in charge of building a new fleet of ships. Each ship requires

40

tons of wood, and accommodates

300

sailors. She receives a delivery of

4

tons of wood each day. The deliveries can continue for

100

days at most, afterwards the weather is too bad to allow them. Overall, she wants to build enough ships to accommodate at least

2100

\newline

How much wood does Astrid need to accommodate

2100

\newline

Posted 10 months ago

Question

Lily's car has a fuel efficiency of

8 \text{ liters}

100 \text{ kilometers}

\newline

What is the fuel efficiency of Lily's car in

\text{kilometers}

\text{liter}

\newline

\frac{\text{km}}{\text{L}}

Posted 10 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant