AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

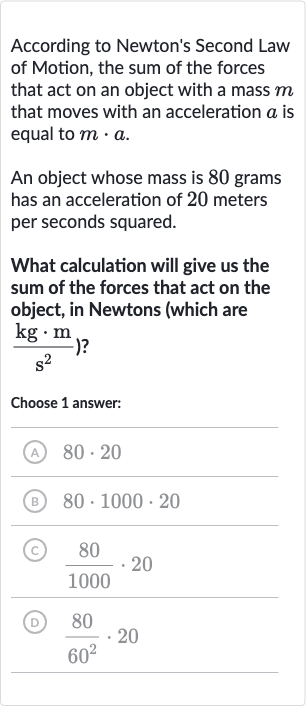
$According to Newton's Second Law of Motion, the sum of the forces that act on an object with a mass m that moves with an acceleration a is equal to m*a. An object whose mass is 80 grams has an acceleration of 20 meters per seconds squared. What calculation will give us the sum of the forces that act on the object, in Newtons (which are {:(kg*m)/(s^(2))) ? Choose 1 answer: (A) 80*20 (B) 80*1000*20 (C) (80)/(1000)*20 (D) (80)/(60^(2))*20$

According to Newton's Second Law of Motion, the sum of the forces that act on an object with a mass $m$ that moves with an acceleration $a$ is equal to $m \cdot a$ . An object whose mass is $80$ grams has an acceleration of $20$ meters per seconds squared. What calculation will give us the sum of the forces that act on the object, in Newtons (which are $\frac{\text{kg} \cdot \text{m}}{\text{s}^2}$ )? $\newline$ Choose $1$ answer: $\newline$ (A) $80 \cdot 20$ $\newline$ (B) $80 \cdot 1000 \cdot 20$ $\newline$ (C) $\frac{80}{1000} \cdot 20$ $\newline$ (D) $\frac{80}{60^2} \cdot 20$

View step-by-step help

Home

Math Problems

Algebra 1

Multi-step problems with unit conversions

Full solution

Q. According to Newton's Second Law of Motion, the sum of the forces that act on an object with a mass

m

that moves with an acceleration

a

is equal to

m \cdot a

. An object whose mass is

80

grams has an acceleration of

20

meters per seconds squared. What calculation will give us the sum of the forces that act on the object, in Newtons (which are

\frac{\text{kg} \cdot \text{m}}{\text{s}^2}

\newline

Choose

1

answer:

\newline

(A)

80 \cdot 20

\newline

(B)

80 \cdot 1000 \cdot 20

\newline

(C)

\frac{80}{1000} \cdot 20

\newline

(D)

\frac{80}{60^2} \cdot 20

Newton's Second Law: Newton's Second Law of Motion states that the force acting on an object is equal to the mass of the object multiplied by its acceleration. The formula is $F = m \times a$ , where $F$ is the force in Newtons, $m$ is the mass in kilograms, and $a$ is the acceleration in meters per second squared.
Convert Mass to Kilograms: The mass of the object is given in grams and needs to be converted to kilograms because the unit for force (Newton) is expressed in kilograms. There are $1000$ grams in a kilogram, so we divide the mass in grams by $1000$ to convert it to kilograms. $\newline$ $80$ grams $= \frac{80}{1000}$ kilograms
Calculate Force: Now we multiply the mass in kilograms by the acceleration to find the force. $\newline$ Force $F = (\frac{80}{1000}) \, \text{kg} \times 20 \, \text{m/s}^2$
Perform Multiplication: Perform the multiplication to calculate the force. $\newline$ $F = (\frac{80}{1000}) \times 20$ $\newline$ $F = 80 \times 20 / 1000$ $\newline$ $F = \frac{1600}{1000}$ $\newline$ $F = 1.6$ Newtons
Correct Calculation: The correct calculation to find the sum of the forces acting on the object is therefore $(80 \, \text{grams})/(1000) \times 20 \, \text{m/s}^2$ , which corresponds to choice (C).