Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

What is the range of this logarithmic function? y=log(x+2) (A) {y∣y >= 10} (B) {y∣y > -2} (C) all real numbers (D) {y∣y > 10} (E) {y∣y >= -2}

What is the range of this logarithmic function?\newliney=log⁡(x+2) y=\log (x+2) \newline(A) {y∣y≥10}\{y \mid y \geq 10\}\newline(B) \{y \mid y>-2\}\newline(C) all real numbers\newline(D) \{y \mid y>10\} \newline(E) {y∣y≥−2}\{y \mid y \geq-2\}

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 1right chevron icon
Domain and range of absolute value functions: equationsright chevron icon

Full solution

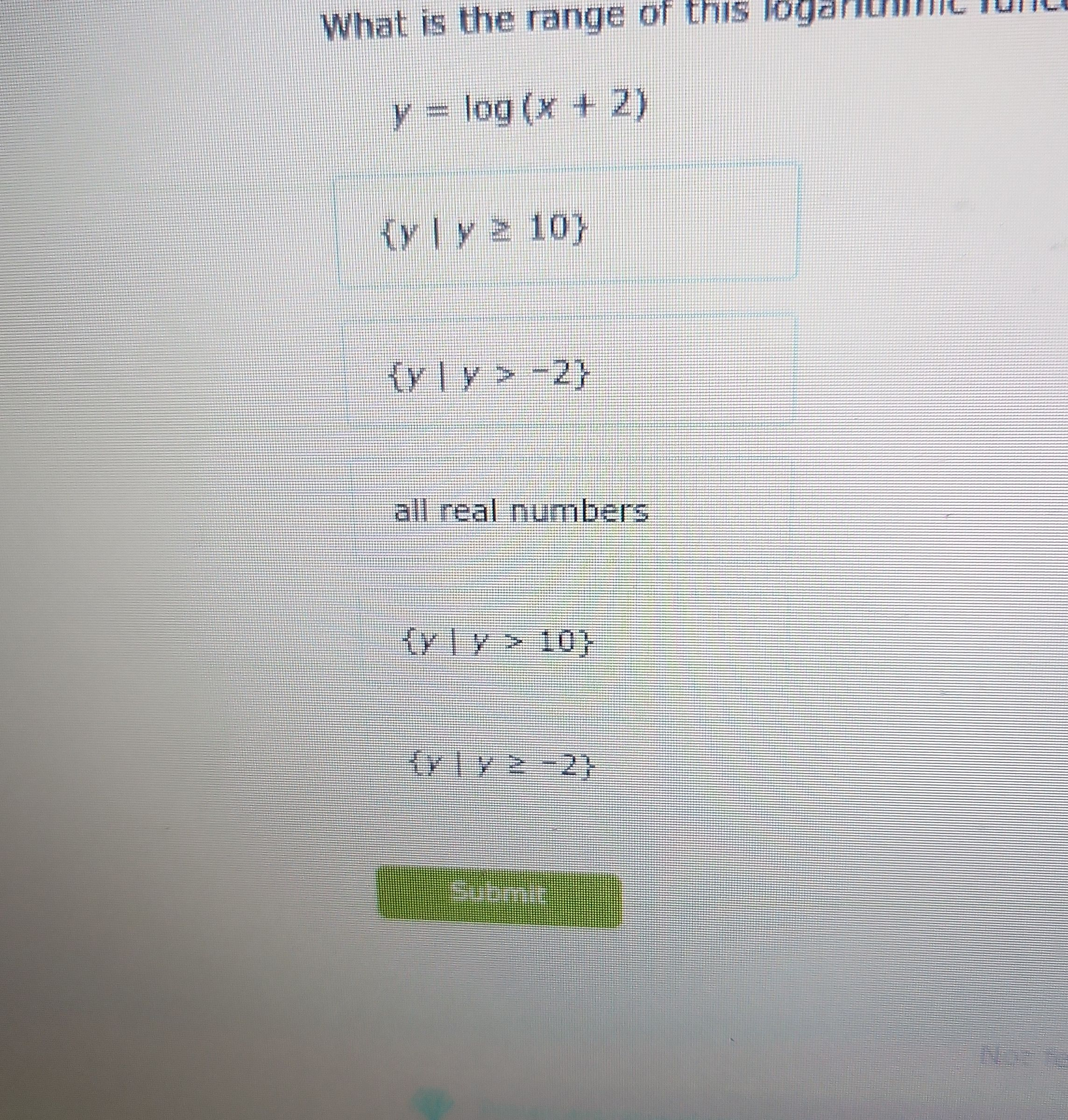
Q. What is the range of this logarithmic function?\newliney=log⁡(x+2) y=\log (x+2) \newline(A) {y∣y≥10}\{y \mid y \geq 10\}\newline(B) {y∣y>−2}\{y \mid y>-2\}\newline(C) all real numbers\newline(D) {y∣y>10}\{y \mid y>10\} \newline(E) {y∣y≥−2}\{y \mid y \geq-2\}
  1. Understand function and question: Understand the function and what is being asked.\newlineThe function given is y=log⁡(x+2)y=\log(x+2), and we are asked to find its range. The range of a function is the set of all possible output values (yy-values) that the function can produce.
  2. Determine domain for function: Determine the domain of the function, as it will affect the range.\newlineThe function y=log⁡(x+2)y=\log(x+2) is defined for all xx such that x+2 > 0, because the logarithm is only defined for positive arguments. Therefore, the domain of the function is x > -2.
  3. Find range using domain: Use the domain to find the range.\newlineSince the logarithm function is continuous and increases without bound as its argument goes to infinity, and since the smallest value in the domain is x=−2x = -2, the smallest value the function can take is log⁡(0)\log(0), which is undefined. As xx increases beyond −2-2, log⁡(x+2)\log(x+2) will take on all values from negative infinity to positive infinity. Therefore, the range of the function is all real numbers.
  4. Check for restrictions or transformations: Check for any restrictions or transformations that could affect the range.\newlineThere are no transformations or restrictions other than the domain limitation, which we have already considered. The logarithm function will continue to increase without bound as xx increases, and will approach negative infinity as xx approaches −2-2 from the right. Therefore, the range remains all real numbers.

More problems from Domain and range of absolute value functions: equations

Question
Find g(x) g(x) , where g(x) g(x) is the translation 2 2 units left of f(x)=∣x∣ f(x) = |x| .\newlineWrite your answer in the form a∣x−h∣+k a|x - h| + k , where a a , h h , and k k are integers.\newlineg(x)= g(x) = ______\newline
Get tutor helpright-arrow

Posted 6 months ago

Question
Find g(x) g(x) , where g(x) g(x) is the reflection across the y y -axis of f(x)=∣x∣ f(x) = |x| .\newlineWrite your answer in the form a∣x−h∣+k a|x - h| + k , where a a , h h , and k k are integers.\newlineg(x)= g(x) = ______\newline
Get tutor helpright-arrow

Posted 10 months ago

Question
The graph of y=∣x∣ y=|x| is reflected across the x x -axis and then scaled vertically by a factor of 77 .\newlineWhat is the equation of the new graph?\newlineChoose 11 answer:\newline(A) y=7∣x∣ y=7|x| \newline(B) y=−7∣x∣ y=-7|x| \newlineC) y=17∣x∣ y=\frac{1}{7}|x| \newline(D) y=−17∣x∣ y=-\frac{1}{7}|x|
Get tutor helpright-arrow

Posted 10 months ago

Question
The graph of y=∣x∣ y=|x| is scaled vertically by a factor of 66 .\newlineWhat is the equation of the new graph?\newlineChoose 11 answer:\newline(A) y=∣x−6∣ y=|x-6| \newline(B) y=6∣x∣ y=6|x| \newline(C) y=16∣x∣ y=\frac{1}{6}|x| \newline(D) y=∣x−16∣ y=\left|x-\frac{1}{6}\right|
Get tutor helpright-arrow

Posted 9 months ago

Question
The graph of y=∣x∣ y=|x| is scaled vertically by a factor of 13 \frac{1}{3} .\newlineWhat is the equation of the new graph?\newlineChoose 11 answer:\newline(A) y=∣x∣−3 y=|x|-3 \newline(B) y=−3∣x∣ y=-3|x| \newline(C) y=13∣x∣ y=\frac{1}{3}|x| \newline(D) y=∣x∣−13 y=|x|-\frac{1}{3}
Get tutor helpright-arrow

Posted 10 months ago

Question
The graph of y=∣x∣ y=|x| is shifted to the left by 66 units.\newlineWhat is the equation of the new graph?\newlineChoose 11 answer:\newline(A) y=∣x+6∣−6 y=|x+6|-6 \newline(B) y=∣x−6∣−6 y=|x-6|-6 \newline(C) y=∣x∣−6 y=|x|-6 \newline(D) y=∣x+6∣ y=|x+6|
Get tutor helpright-arrow

Posted 9 months ago

Question
The graph of y=∣x∣ y=|x| is reflected across the x x -axis and then scaled vertically by a factor of 14 \frac{1}{4} .\newlineWhat is the equation of the new graph?\newlineChoose 11 answer:\newline(A) y=−4∣x∣ y=-4|x| \newline(B) y=∣x∣−4 y=|x|-4 \newline(C) y=−14∣x∣ y=-\frac{1}{4}|x| \newline(D) y=∣x−4∣ y=|x-4|
Get tutor helpright-arrow

Posted 10 months ago

Question
The graph of y=∣x∣ y=|x| is shifted down by 99 units.\newlineWhat is the equation of the new graph?\newlineChoose 11 answer:\newline(A) y=∣x∣−9 y=|x|-9 \newline(B) y=∣x+9∣ y=|x+9| \newline(C) y=∣x∣+9 y=|x|+9 \newline(D) y=∣x−9∣ y=|x-9|
Get tutor helpright-arrow

Posted 1 year ago

Question
y=13x+5y=2x \begin{array}{l} y=\frac{1}{3} x+5 \\ y=2 x \end{array} \newlineConsider the given system of equations. If (x,y) (x, y) is the solution to the system, then what is the value of y+x y+x ?
Get tutor helpright-arrow

Posted 5 months ago

Question
13x+3y=4 \frac{1}{3} x+3 y=4 \newline2x−4=2y 2 x-4=2 y \newlineConsider the given system of equations. If (x,y) (x, y) is the solution to the system, then what is the value of x−y x-y ?
Get tutor helpright-arrow

Posted 4 months ago

View all (19)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant