Full solution
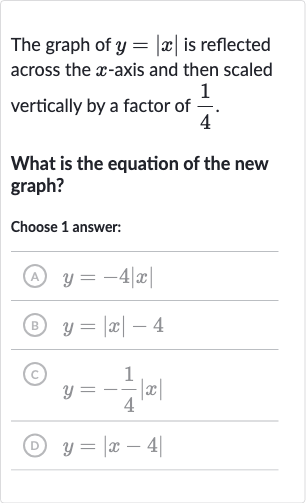
Q. The graph of is reflected across the -axis and then scaled vertically by a factor of .What is the equation of the new graph?Choose answer:(A) (B) (C) (D)
- Reflect across x-axis: Reflect across the x-axis.To reflect a graph across the x-axis, we multiply the function by .Reflected function:
- Scale vertically by : Scale the reflected function vertically by a factor of . To scale a function vertically, we multiply the function by the scaling factor. Scaled function: \(y = \left(\frac{\(1\)}{\(4\)}\right)(-|x|) = -\left(\frac{\(1\)}{\(4\)}\right)|x|
More problems from Transformations of absolute value functions: translations and reflections
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help