Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

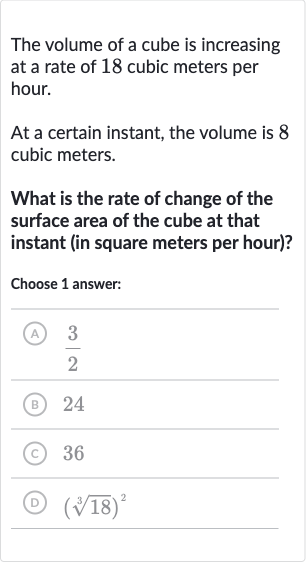
The volume of a cube is increasing at a rate of 18 cubic meters per hour. At a certain instant, the volume is 8 cubic meters. What is the rate of change of the surface area of the cube at that instant (in square meters per hour)? Choose 1 answer: (A) (3)/(2) (B) 24 (C) 36 (D) (root(3)(18))^(2)

The volume of a cube is increasing at a rate of 1818 cubic meters per hour.\newlineAt a certain instant, the volume is 88 cubic meters.\newlineWhat is the rate of change of the surface area of the cube at that instant (in square meters per hour)?\newlineChoose 11 answer:\newline(A) 32 \frac{3}{2} \newline(B) 2424\newline(C) 3636\newline(D) (183)2 (\sqrt[3]{18})^{2}

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Grade 6right chevron icon
Area of quadrilaterals and triangles: word problemsright chevron icon

Full solution

Q. The volume of a cube is increasing at a rate of 1818 cubic meters per hour.\newlineAt a certain instant, the volume is 88 cubic meters.\newlineWhat is the rate of change of the surface area of the cube at that instant (in square meters per hour)?\newlineChoose 11 answer:\newline(A) 32 \frac{3}{2} \newline(B) 2424\newline(C) 3636\newline(D) (183)2 (\sqrt[3]{18})^{2}
  1. Find side length: First, find the side length of the cube when the volume is 88 cubic meters. The formula for the volume of a cube is V=s3V = s^3, where ss is the side length.
  2. Calculate side length: Calculate the side length: 8=s38 = s^3. So, s=83s = \sqrt[3]{8}, which is 22 meters.
  3. Find surface area: Now, find the surface area of the cube using the side length. The formula for the surface area of a cube is SA=6s2SA = 6s^2.
  4. Calculate surface area: Calculate the surface area: SA=6×(22)=6×4=24SA = 6 \times (2^2) = 6 \times 4 = 24 square meters.
  5. Find rate of change: Next, find the rate of change of the side length as the volume increases. The formula for the volume of a cube is V=s3V = s^3, so dVdt=3s2⋅dsdt\frac{dV}{dt} = 3s^2 \cdot \frac{ds}{dt}, where dVdt\frac{dV}{dt} is the rate of change of volume and dsdt\frac{ds}{dt} is the rate of change of the side length.
  6. Calculate rate of change: Plug in the known values: 18=3×(22)×dsdt18 = 3 \times (2^2) \times \frac{ds}{dt}. So, 18=3×4×dsdt18 = 3 \times 4 \times \frac{ds}{dt}, which means dsdt=1812=1.5\frac{ds}{dt} = \frac{18}{12} = 1.5 meters per hour.
  7. Find rate of change: Finally, find the rate of change of the surface area. The formula for the surface area of a cube is SA=6s2SA = 6s^2, so dSAdt=6×2s×dsdt.\frac{dSA}{dt} = 6 \times 2s \times \frac{ds}{dt}.
  8. Calculate rate of change: Plug in the known values: dSAdt=6×2×2×1.5\frac{dSA}{dt} = 6 \times 2 \times 2 \times 1.5. So, dSAdt=6×4×1.5=36\frac{dSA}{dt} = 6 \times 4 \times 1.5 = 36 square meters per hour.
  9. Final answer: The correct answer is (C) 3636 square meters per hour.

More problems from Area of quadrilaterals and triangles: word problems

Question
The work done by stretching a certain spring increases by 0.13x 0.13 x joules per centimeter (where x x is the displacement, in centimeters, beyond the spring's natural length).\newlineHow much work (in Joules) must be done in order to stretch the spring from x=4 x=4 to x=10 x=10 ?\newlineChoose 11 answer:\newline(A) 55.4646\newline(B) 77.5454\newline(C) 10.92 \mathbf{1 0 . 9 2} \newline(D) 15.08 \mathbf{1 5 . 0 8}
Get tutor helpright-arrow

Posted 9 months ago

Question
Ahmed is modeling the area covered by a moss using the following equation:\newlineA=t2+5.8t+9.41 A=t^{2}+5.8 t+9.41 \newlinewhere A A is the area covered in square centimeters, negative values of t t represent a number of days before noon on July 11 , 20142014 , and positive values of t t represent a number of days after noon on July 11,20142014 . Which of the following equivalent expressions contains the number of days before noon on July 11,20142014 , as a constant or coefficient, when the moss started with its smallest area of 11 square centimeter?\newlineChoose 11 answer:\newline(A) (t−(−2.9))2+1 (t-(-2.9))^{2}+1 \newline(B) (t−(−1))2+3.8t+8.41 (t-(-1))^{2}+3.8 t+8.41 \newline(C) (t−(−3.9))(t−(−1.9))+2 (t-(-3.9))(t-(-1.9))+2 \newline(D) t(t−(−5.8))+9.41 t(t-(-5.8))+9.41
Get tutor helpright-arrow

Posted 9 months ago

Question
Solve.\newlineFind the exact volume and surface area of a sphere of radius 1212 inches.\newlineV=576π cu in.;V=576\pi\text{ cu in.}; SA=2304π sq in.SA=2304\pi\text{ sq in.}\newlineV=2304π cu in.;V=2304\pi\text{ cu in.}; SA=576π sq in.SA=576\pi\text{ sq in.}\newlineV=1728π cu inV=1728\pi\text{ cu in} ;SA=144π sq in.;SA=144\pi\text{ sq in.}\newlineV=6912π cuin.:V=6912\pi\text{ cuin.}:SA⋅=36π sq in.SA^{\cdot}=36\pi\text{ sq in.}\newline
Get tutor helpright-arrow

Posted 8 months ago

Question
What is the height of the tent?
Get tutor helpright-arrow

Posted 8 months ago

Question
The volume of a rectangular prism is 108 cm3 108 \mathrm{~cm}^{3} . Eric measures the sides to be 3.32 cm 3.32 \mathrm{~cm} by 4.04 cm 4.04 \mathrm{~cm} by 8.72 cm 8.72 \mathrm{~cm} . In calculating the volume, what is the relative error, to the nearest thousandth.\newlineAnswer:
Get tutor helpright-arrow

Posted 8 months ago

Question
In △UVW,w=8.9 \triangle \mathrm{UVW}, w=8.9 inches, v=9 v=9 inches and ∠V=50∘ \angle \mathrm{V}=50^{\circ} . Find all possible values of ∠W \angle \mathrm{W} , to the nearest 1010th of a degree.\newlineAnswer:
Get tutor helpright-arrow

Posted 8 months ago

Question
Circle O O shown below has a radius of 77 inches. To the nearest tenth of an inch, determine the length of the arc, x x , subtended by an angle of 11.44 radians.\newlineAnswer: □\square inches
Get tutor helpright-arrow

Posted 8 months ago

Question
Circle O O shown below has an arc of length 1919 inches subtended by an angle of 11 radians. Find the length of the radius, x x , to the nearest tenth of an inch.\newlineAnswer: □\square inches
Get tutor helpright-arrow

Posted 8 months ago

Question
The area of a rectangular goat pen is 54 square meters54 \, \text{square meters}. The perimeter is 30 meters30 \, \text{meters}. What are the dimensions of the pen?\newline____\_\_\_\_ meters by ____\_\_\_\_ meters
Get tutor helpright-arrow

Posted 8 months ago

Question
The perimeter of a square piece of tissue paper is 3636 centimeters. How long is each side of the tissue paper?\newline​_____ centimeters
Get tutor helpright-arrow

Posted 8 months ago

View all (25)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant