Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

The following are all angle measures (in radians, rounded to the nearest hundredth) whose tangent is -1.4 .
Which is the principal value of
tan^(-1)(-1.4) ?
Choose 1 answer:
(A) -4.09
(B) -0.95
(C)
2.19
(D) 5.33

The following are all angle measures (in radians, rounded to the nearest hundredth) whose tangent is $-1$ . $4$ . $\newline$ Which is the principal value of $\tan ^{-1}(-1.4) ?$ $\newline$ Choose $1$ answer: $\newline$ (A) $\quad-4.09$ $\newline$ (B) $-0$ . $95$ $\newline$ (C) $\mathbf{2 . 1 9}$ $\newline$ (D) $5$ . $33$

View step-by-step help

View step-by-step help

Calculate mean absolute deviation

Full solution

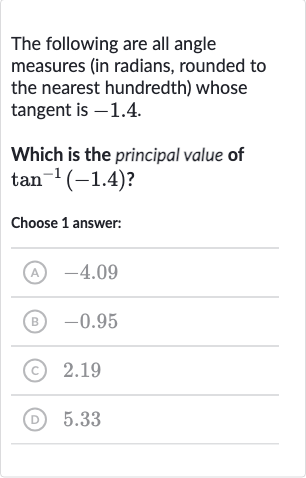
Q. The following are all angle measures (in radians, rounded to the nearest hundredth) whose tangent is

-1

.

4

.

\newline

Which is the principal value of

\tan ^{-1}(-1.4) ?

\newline

Choose

1

answer:

\newline

(A)

\quad-4.09

\newline

(B)

-0

.

95

\newline

(C)

\mathbf{2 . 1 9}

\newline

(D)

5

.

33

Determine angle in radians: To find the principal value of $\tan^{-1}(-1.4)$ , we need to determine the angle in radians whose tangent is $-1.4$ and lies in the range of the inverse tangent function, which is between $-\pi/2$ and $\pi/2$ .
Calculate inverse tangent: Using a calculator or inverse tangent function, we calculate $\tan^{-1}(-1.4)$ . The calculator should be set to radians mode for this calculation.
Obtain calculated value: The calculator gives us the value of $\tan^{-1}(-1.4)$ as approximately $-0.9505$ radians when rounded to the nearest hundredth.
Compare with options: Comparing the calculated value with the options given, we find that option (B) $-0.95$ is the closest to our calculated value and falls within the range of the inverse tangent function.
Identify principal value: Therefore, the principal value of $\tan^{-1}(-1.4)$ is $-0.95$ radians, which corresponds to option $(B)$ .

More problems from Calculate mean absolute deviation

Question

In the data set below, what is the mean absolute deviation?

\newline

6,3,1,6,6

\newline

If the answer is a decimal, round it to the nearest tenth.

\newline

mean absolute deviation (MAD): _____

Posted 2 months ago

Question

Look at this set of

8

60, 38, 93, 14, 23, 81, 12, 64

, How would the range change if the number

18

replaced the number

12

\newline

\newline

\newline

\newline

Posted 1 month ago

Question

Liam has the following data:

20,7,x,20

. If the mean is

14

, which number could

x

\newline

\newline

(A)9

\newline

(B)21

Posted 2 months ago

Question

g=15-\frac{m}{32}

\newline

If Vera starts with a full tank of gas, the number of gallons of gas,

g

, left in the tank after driving

m

miles is given by the equation. When full, how many gallons of gas does Vera's tank hold?

\newline

1

\newline

15

\newline

17

\newline

32

\newline

480

Posted 4 months ago

Question

x-3 y=8

\newline

y^{2}=x-4 y-2

\newline

(x, y)

is a solution to the system of equations shown, which of the following are

y

-coordinates of the solutions?

\newline

1

\newline

-1

-3

\newline

14

2

\newline

-2

3

\newline

2

-3

Posted 2 months ago

Question

Which of the following expressions is equivalent to

-2 x y+18

\newline

1

\newline

-2(x y-9)

\newline

-2(x y+9)

\newline

2(x y-9)

\newline

2(x y+9)

Posted 1 month ago

Question

What is the value of

\tan \left(\frac{\pi}{3}\right)

\newline

1

\newline

\frac{1}{2}

\newline

\frac{\sqrt{3}}{3}

\newline

\frac{\sqrt{3}}{2}

\newline

\sqrt{3}

Posted 4 months ago

Question

Which of the following is the value of

\tan (\pi)

\newline

1

\newline

1

\newline

0

\newline

-1

\newline

\tan (\pi)

Posted 4 months ago

Question

Which of the following is the value of

\sin \left(\frac{\pi}{2}\right) ?

\newline

1

\newline

-1

\newline

0

\newline

\frac{\sqrt{2}}{2}

\newline

1

Posted 4 months ago

Question

\begin{array}{l} 3 x+y=3 \\ 7 x-y=2 \end{array}

\newline

The given system of equations has solution

(x, y)

. What is the value of

x

Posted 1 month ago