Full solution
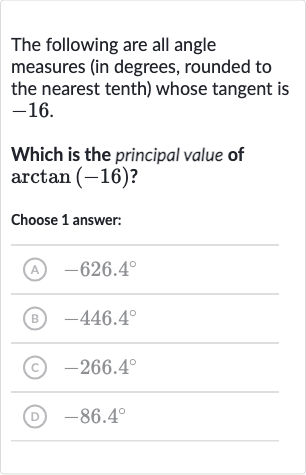
Q. The following are all angle measures (in degrees, rounded to the nearest tenth) whose tangent is .Which is the principal value of ?Choose answer:(A) (B) (C) (D)
- Principal Value Range: The principal value of an arctan function is the value of the inverse tangent that lies between degrees and degrees.
- Calculate : To find the principal value of , we can use a calculator or a computer to compute the inverse tangent of .
- Use Calculator: Using a calculator, we find that is approximately degrees.
- Verify Principal Value: The principal value of must be in the range of degrees to degrees, and since degrees falls within this range, it is the correct principal value.
More problems from Midpoint formula: find the midpoint
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help