Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Find the sum of the first 7 terms in the following geometric series. Do not round your answer. 1024+512+256+dots

Find the sum of the first 77 terms in the following geometric series. Do not round your answer.\newline1024+512+256+… 1024+512+256+\ldots

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 1right chevron icon
Distance between two pointsright chevron icon

Full solution

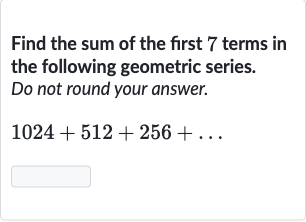
Q. Find the sum of the first 77 terms in the following geometric series. Do not round your answer.\newline1024+512+256+… 1024+512+256+\ldots
  1. Identify first term and common ratio: To find the sum of the first 77 terms of a geometric series, we need to identify the first term (a1a_1) and the common ratio (rr) of the series.\newlineThe first term a1a_1 is 10241024.\newlineTo find the common ratio, we divide the second term by the first term: r=5121024=12r = \frac{512}{1024} = \frac{1}{2}.
  2. Calculate the common ratio: Now that we have the first term and the common ratio, we can use the formula for the sum of the first nn terms of a geometric series: Sn=a1×(1−rn)/(1−r)S_n = a_1 \times (1 - r^n) / (1 - r), where nn is the number of terms.\newlineWe want to find the sum of the first 77 terms, so n=7n = 7.
  3. Use the formula for sum of first n terms: Let's plug the values into the formula: S7=1024×(1−(1/2)7)/(1−1/2)S_7 = 1024 \times (1 - (1/2)^7) / (1 - 1/2).\newlineNow we calculate (1/2)7(1/2)^7.
  4. Plug in values into the formula: (12)7=1128(\frac{1}{2})^7 = \frac{1}{128}.\newlineNow we substitute this value back into the sum formula: S7=1024×(1−1128)/(1−12)S_7 = 1024 \times (1 - \frac{1}{128}) / (1 - \frac{1}{2}).
  5. Calculate (1/2)7(1/2)^7: Simplify the expression inside the parentheses: 1−1/128=127/1281 - 1/128 = 127/128. Now the sum formula looks like this: S7=1024×(127/128)/(1/2)S_7 = 1024 \times (127/128) / (1/2).
  6. Substitute value into sum formula: We simplify the denominator: 1−12=121 - \frac{1}{2} = \frac{1}{2}. Now we have S7=1024×(127128)/(12)S_7 = 1024 \times \left(\frac{127}{128}\right) / \left(\frac{1}{2}\right).
  7. Simplify expression inside parentheses: To divide by 12\frac{1}{2}, we multiply by the reciprocal, which is 22. So, S7=1024×(127128)×2S_7 = 1024 \times \left(\frac{127}{128}\right) \times 2.
  8. Simplify the denominator: Now we perform the multiplication: 1024×2=20481024 \times 2 = 2048. Then we multiply this by 127/128127/128: S7=2048×(127/128)S_7 = 2048 \times (127/128).
  9. Multiply by reciprocal: Finally, we calculate the multiplication: 2048×(127/128)=2048×127/1282048 \times (127/128) = 2048 \times 127 / 128.
  10. Perform multiplication: Perform the division: 2048×127/128=2048/128×1272048 \times 127 / 128 = 2048 / 128 \times 127.\newline2048/128=162048 / 128 = 16.\newlineSo, S7=16×127S_7 = 16 \times 127.
  11. Perform division: Now we multiply 1616 by 127127 to get the final sum: S7=16×127=2032S_7 = 16 \times 127 = 2032.

More problems from Distance between two points

Question
Find the sum of the first 99 terms in the following geometric series.\newlineDo not round your answer.\newline64+32+16+… 64+32+16+\ldots
Get tutor helpright-arrow

Posted 9 months ago

Question
Vector u⃗ \vec{u} has an initial point (−5,−8) (-5,-8) and a terminal point (6,2) (6,2) .\newlineFind the components of vector u⃗ \vec{u} .\newlineu⃗=(□,□) \vec{u}=(\square, \square)
Get tutor helpright-arrow

Posted 8 months ago

Question
Vector b⃗ \vec{b} has an initial point (−2,7) (-2,7) and a terminal point (9,4) (9,4) .\newlineFind the components of vector b⃗ \vec{b} .\newlineb⃗=□,□) \vec{b}=\square, \square)
Get tutor helpright-arrow

Posted 9 months ago

Question
The following are all angle measures (in degrees, rounded to the nearest tenth) whose tangent is 22.66 .\newlineWhich is the principal value of arctan⁡(2.6) \arctan (2.6) ?\newlineChoose 11 answer:\newline(A) −111.0∘ -111.0^{\circ} \newline(B) 69.0∘ 69.0^{\circ} \newline(C) 249.0∘ 249.0^{\circ} \newline(D) 429.0∘ 429.0^{\circ}
Get tutor helpright-arrow

Posted 9 months ago

Question
The following are all angle measures (in degrees, rounded to the nearest tenth) whose tangent is −16-16 .\newlineWhich is the principal value of arctan⁡(−16) \arctan (-16) ?\newlineChoose 11 answer:\newline(A) −626.4∘ -626.4^{\circ} \newline(B) −446.4∘ -446.4^{\circ} \newline(C) −266.4∘ -266.4^{\circ} \newline(D) −86.4∘ -86.4^{\circ}
Get tutor helpright-arrow

Posted 9 months ago

Question
Use the distributive property to write an equivalent expression.\newline4(f−2g+1) 4(f-2 g+1) \newlineAnswer:
Get tutor helpright-arrow

Posted 8 months ago

Question
Use the distributive property to write an equivalent expression.\newline9(g+3) 9(g+3) \newlineAnswer:
Get tutor helpright-arrow

Posted 8 months ago

Question
Use the distributive property to write an equivalent expression.\newline3(2n+10p−4) 3(2 n+10 p-4) \newlineAnswer:
Get tutor helpright-arrow

Posted 8 months ago

Question
Use the distributive property to write an equivalent expression.\newline4(4k−7m+10) 4(4 k-7 m+10) \newlineAnswer:
Get tutor helpright-arrow

Posted 8 months ago

Question
You pick a card at random, put it back, and then pick another card at random.\newline44\newline55\newline66\newline77\newlineWhat is the probability of picking a number greater than 55 and then picking a 44 ?\newlineWrite your answer as a percentage.
Get tutor helpright-arrow

Posted 1 month ago

View all (140)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant