Full solution
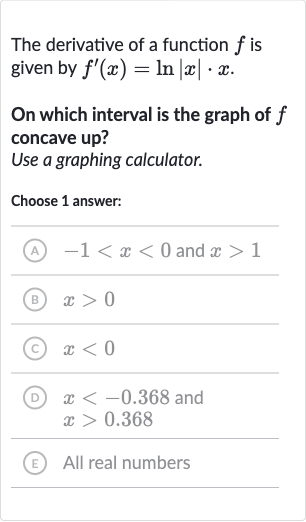
Q. The derivative of a function is given by .On which interval is the graph of concave up?Use a graphing calculator.Choose answer:(A) and (B) (C) (D) and (E) All real numbers
- Calculate First Derivative: To determine where the graph of is concave up, we need to find the second derivative, , and look for where it is positive.
- Apply Product Rule: Use the product rule for differentiation: ' = u'v + uv'. Let and . Then and .
- Simplify Second Derivative: Simplify the second derivative:
- Determine Positive Values: Find where is positive: 1 + \ln|x| > 0 \ln|x| > -1
- Solve for x (x > 0): Solve for x in the inequality \ln|x| > -1. For x > 0: \ln(x) > -1 For x < 0: \ln(-x) > -1
- Solve for x x < 0: Exponentiate both sides of the inequalities to solve for x.For x > 0: e^{\ln(x)} > e^{-1}, so x > \frac{1}{e}For x < 0: e^{\ln(-x)} > e^{-1}, so -x > \frac{1}{e}, which means x < -\frac{1}{e}