AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
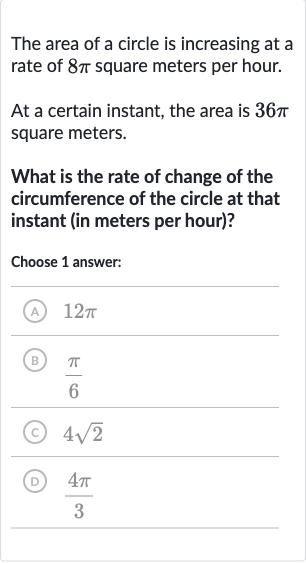
The area of a circle is increasing at a rate of square meters per hour.At a certain instant, the area is square meters.What is the rate of change of the circumference of the circle at that instant (in meters per hour)?Choose answer:(A) (B) (C) (D)
Full solution
Q. The area of a circle is increasing at a rate of square meters per hour.At a certain instant, the area is square meters.What is the rate of change of the circumference of the circle at that instant (in meters per hour)?Choose answer:(A) (B) (C) (D)
- Remember Circle Area Formula: First, let's remember the formula for the area of a circle, , where is the radius.
- Find Radius from Area: Given that the area is square meters, we can find the radius by rearranging the formula: . So, .
- Calculate Circumference: Now, we take the square root of both sides to find . meters.
- Differentiate Circumference: The formula for the circumference of a circle is . So, the circumference at that instant is meters.
- Rate of Area Change: To find the rate of change of the circumference, we need to differentiate the circumference with respect to time. .
- Solve for : We know the area is increasing at a rate of square meters per hour, so . Since , then .
- Substitute into Circumference Rate: We can now solve for : . So, meters per hour.
- Substitute into Circumference Rate: We can now solve for : . So, meters per hour.Finally, we substitute back into the rate of change of the circumference: meters per hour.
More problems from Area of quadrilaterals and triangles: word problems
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
