Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

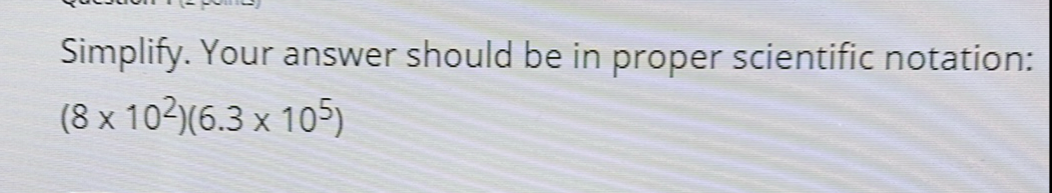
Simplify. Your answer should be in proper scientific notation:

(8×10^(2))(6.3 ×10^(5))

Simplify. Your answer should be in proper scientific notation: $\newline$ $\left(8 \times 10^{2}\right)\left(6.3 \times 10^{5}\right)$

View step-by-step help

View step-by-step help

Add and subtract numbers written in scientific notation

Full solution

Q. Simplify. Your answer should be in proper scientific notation:

\newline

\left(8 \times 10^{2}\right)\left(6.3 \times 10^{5}\right)

Multiply Coefficients: Multiply the coefficients ( $8$ and $6.3$ ). $\newline$ To find the product of two numbers in scientific notation, first multiply their coefficients. $\newline$ Calculation: $8 \times 6.3 = 50.4$
Add Exponents: Add the exponents ( $2$ and $5$ ). $\newline$ When multiplying powers of $10$ , you add the exponents. $\newline$ Calculation: $10^2 \times 10^5 = 10^{2+5} = 10^7$
Combine Results: Combine the results from Step $1$ and Step $2$ to write the product in scientific notation. $\newline$ The product of the coefficients is $50.4$ , and the combined exponent of $10$ is $7$ . $\newline$ However, proper scientific notation requires the coefficient to be between $1$ and $10$ . Since $50.4$ is not in this range, we need to adjust it. $\newline$ Calculation: $50.4 \times 10^7$ can be rewritten as $(5.04 \times 10) \times 10^7$
Adjust Coefficient: Adjust the coefficient to proper scientific notation by factoring out a power of $10$ . $\newline$ We can rewrite $5.04 \times 10$ as $5.04 \times 10^1$ . $\newline$ Calculation: $5.04 \times 10^1 \times 10^7 = 5.04 \times 10^{1+7} = 5.04 \times 10^8$

More problems from Add and subtract numbers written in scientific notation

Question

16 a+72

to identify the equivalent expressions.

\newline

2

\newline

4(4 a+18)

\newline

8(2 a+9)

\newline

2(8+36 a)

\newline

2(8 a+72)

Posted 1 year ago

Question

30-6 v-18 w

to identify the equivalent expressions.

\newline

2

\newline

2(15-12 v)

\newline

3(10-3 v+6 w)

\newline

6(5-v-3 w)

\newline

2(15-3 v-9 w)

Posted 1 year ago

Question

24 m-12 p+72

to identify the equivalent expressions.

\newline

2

\newline

6(4 m+2 p+12)

\newline

2(12 m-6 p+36)

\newline

12(2 m-p+6)

\newline

24(m-12 p+3)

Posted 1 year ago

Question

48-8 x

to identify the equivalent expressions.

\newline

2

\newline

3(16-8 x)

\newline

2(24-4 x)

\newline

8(6-x)

\newline

4(12-4 x)

Posted 1 year ago

Question

Multiply and simplify the following complex numbers:

\newline

(-3-2 i) \cdot(-4+2 i)

Posted 1 year ago

Question

Multiply and simplify the following complex numbers:

\newline

(1-2 i) \cdot(4+i)

Posted 1 year ago

Question

Multiply and simplify the following complex numbers:

\newline

(-1+4 i) \cdot(4-3 i)

Posted 1 year ago

Question

Multiply and simplify the following complex numbers:

\newline

(-4-4 i) \cdot(-5-3 i)

Posted 1 year ago

Question

Multiply and simplify the following complex numbers:

\newline

(1+5 i) \cdot(-3-i)

Posted 1 year ago

Question

Multiply and simplify the following complex numbers:

\newline

(-2+2 i) \cdot(5+5 i)

Posted 1 year ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant