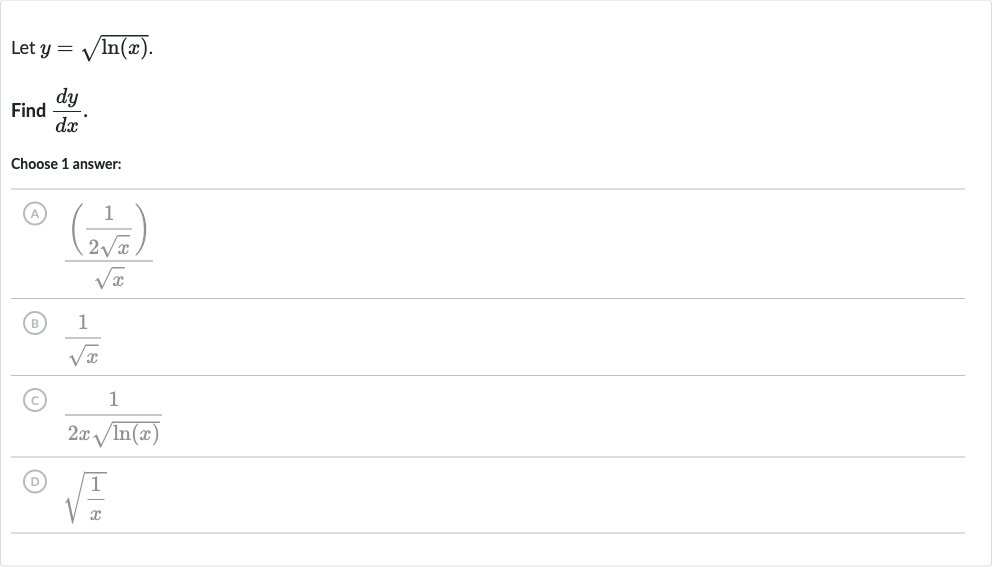
Let y=sqrt(ln(x)). Find (dy)/(dx). Choose 1 answer: (A) (((1)/(2sqrtx)))/(sqrtx) (B) (1)/(sqrtx) (C) (1)/(2xsqrt(ln(x))) (D) sqrt((1)/(x))

Question

Let  y=sqrt(ln(x)). Find  (dy)/(dx). Choose 1 answer: (A)  (((1)/(2sqrtx)))/(sqrtx) (B)  (1)/(sqrtx) (C)  (1)/(2xsqrt(ln(x))) (D)  sqrt((1)/(x))

Bytelearn · Accepted Answer

Identify Function: Identify the function to differentiate. We have the function y = sqrt(ln(x)). We need to find the derivative of y with respect to x, which is denoted as (dy)/(dx).Apply Chain Rule: Apply the chain rule for differentiation. The chain rule states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function. In this case, the outer function is the square root function, and the inner function is the natural logarithm of x.Differentiate Outer Function: Differentiate the outer function with respect to the inner function. The outer function is sqrt(u), where u = ln(x). The derivative of sqrt(u) with respect to u is (1)/(2sqrt(u)). We will substitute u back with ln(x) later.Differentiate Inner Function: Differentiate the inner function with respect to x. The inner function is ln(x). The derivative of ln(x) with respect to x is (1)/(x).Apply Chain Rule: Apply the chain rule by multiplying the derivatives from Step 3 and Step 4. (dy)/(dx) = (1)/(2sqrt(ln(x))) * (1)/(x) = (1)/(2x*sqrt(ln(x))).Match Result: Match the result with the given choices. The derivative we found is (1)/(2x*sqrt(ln(x))), which corresponds to choice (C).

Let $y=\sqrt{\ln (x)}$ . $\newline$ Find $\frac{d y}{d x}$ . $\newline$ Choose $1$ answer: $\newline$ (A) $\frac{\left(\frac{1}{2 \sqrt{x}}\right)}{\sqrt{x}}$ $\newline$ (B) $\frac{1}{\sqrt{x}}$ $\newline$ (C) $\frac{1}{2 x \sqrt{\ln (x)}}$ $\newline$ (D) $\sqrt{\frac{1}{x}}$

Full solution

More problems from Domain and range of square root functions: equations

Related topics