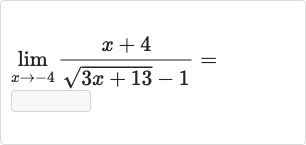lim_(x rarr-4)(x+4)/(sqrt(3x+13)-1)=

Question

Bytelearn · Accepted Answer

Identify Function & Point: Identify the function and the point at which we need to find the limit. We have the function f(x) = (x+4)/(sqrt(3x+13)-1) and we need to find the limit as x approaches -4.Substitute x = -4: Substitute x = -4 into the function to see if the limit can be directly calculated. lim_(x -> -4) (x+4)/(sqrt(3x+13)-1) = (-4+4)/(sqrt(3(-4)+13)-1) = 0/(sqrt(-12+13)-1) = 0/0 We encounter an indeterminate form 0/0, which means we cannot directly calculate the limit.Apply Algebraic Manipulation: Apply algebraic manipulation to eliminate the indeterminate form. We can multiply the numerator and the denominator by the conjugate of the denominator to rationalize it. The conjugate of sqrt(3x+13)-1 is sqrt(3x+13)+1.Multiply by Conjugate: Multiply the function by the conjugate over itself. lim_(x -> -4) [(x+4)(sqrt(3x+13)+1)] / [(sqrt(3x+13)-1)(sqrt(3x+13)+1)]Apply Difference of Squares: Apply the difference of squares formula to the denominator. The difference of squares formula is (a-b)(a+b) = a^2 - b^2. lim_(x -> -4) [(x+4)(sqrt(3x+13)+1)] / [(3x+13) - (1)^2]Simplify Denominator: Simplify the denominator. lim_(x -> -4) [(x+4)(sqrt(3x+13)+1)] / [3x+13 - 1] lim_(x -> -4) [(x+4)(sqrt(3x+13)+1)] / [3x+12]Cancel Common Factors: Cancel out the common factor of (x+4) in the numerator and the factor of 3(x+4) in the denominator. lim_(x -> -4) [sqrt(3x+13)+1] / 3Substitute x = -4: Substitute x = -4 into the simplified function to find the limit. lim_(x -> -4) [sqrt(3(-4)+13)+1] / 3 = [sqrt(-12+13)+1] / 3 = [sqrt(1)+1] / 3 = (1+1) / 3 = 2/3

$\lim _{x \rightarrow-4} \frac{x+4}{\sqrt{3 x+13}-1}=$

Full solution

More problems from Domain and range of square root functions: equations

Related topics

lim⁡x→−4x+43x+13−1= \lim _{x \rightarrow-4} \frac{x+4}{\sqrt{3 x+13}-1}= limx→−4​3x+13​−1x+4​=

$\lim _{x \rightarrow-4} \frac{x+4}{\sqrt{3 x+13}-1}=$