AI tutor
Full solution
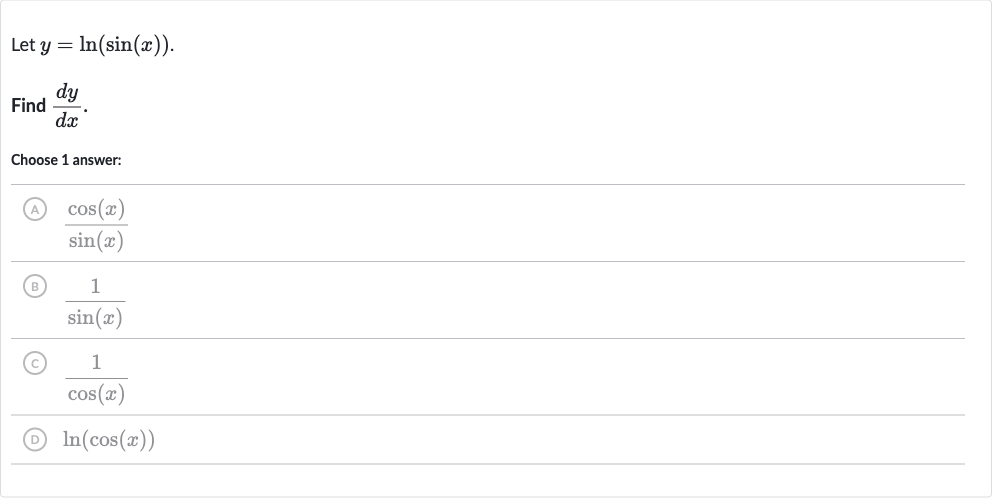
Q. Let .Find .Choose answer:(A) (B) (C) (D)
- Identify Function: Identify the function and its composition.We have , which is a composition of two functions: the natural logarithm function and the sine function. We need to find the derivative of this composite function.
- Apply Chain Rule: Apply the chain rule for differentiation. The chain rule states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function. In this case, the outer function is (where ) and the inner function is .
- Differentiate Outer Function: Differentiate the outer function with respect to the inner function.The derivative of with respect to is . So, when we apply this to our function, we get the derivative of with respect to as .
- Differentiate Inner Function: Differentiate the inner function with respect to . The derivative of with respect to is . So, we will multiply the result from Step by .
- Combine Results: Combine the results from Steps and .Multiplying by , we get , which can also be written as .
- Match Final Result: Match the final result with the given options.The derivative is , which matches option (A).