AI tutor
Full solution
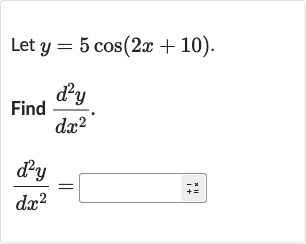
Q. Let .Find .
- Find First Derivative: We are given the function , and we need to find the second derivative of with respect to , which is denoted as . The first step is to find the first derivative of with respect to . Using the chain rule, the derivative of with respect to is , where in this case.
- Find Second Derivative: Now that we have the first derivative, we need to find the second derivative. We will differentiate with respect to . Using the chain rule again, the derivative of with respect to is , where .
- Simplify Expression: Simplify the expression for the second derivative.
More problems from Evaluate expression when two complex numbers are given
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help